Difference between revisions of "2735: Coordinate Plane Closure"
(→Transcript: complete) |
|||
| (14 intermediate revisions by 14 users not shown) | |||
| Line 10: | Line 10: | ||
==Explanation== | ==Explanation== | ||
| − | {{ | + | This comic is a "Math Notice," which is presumably a warning or reminder for mathematicians or others who interact with the field of mathematics, in a similar way to how a "Travel Notice" may prewarn drivers of planned road closures for repairs (or [https://www.cameroncountytx.gov/spacex/ rocketry]). It also has similarities to that of a {{w|Notice to mariners}} or {{w|NOTAM|air missions}}, where nautical or aeronautical navigation might be impinged by an area (or volume) that should be kept clear from in the near future, and to notices from websites or software providers about planned maintenance, which alert users about upcoming outages. Specifically, this notice advises those who are using the coordinate plane to avoid drawing any graphs in the area with a hole until the damage is patched or fixed. |
| − | + | The joke may have been inspired as a response to the {{w|2023 China balloon incident}}, which occurred a few days earlier. This had occasioned one of the largest temporary flight restrictions, with a closed airspace as a response, in U.S. history. | |
{{w|Coordinate planes}} are used in math for drawing graphs. The joke here is that a small section has been "closed for maintenance," likening the concept of a coordinate plane to an actual physical platform used by math, which is therefore vulnerable to damage such as is shown in the comic. In reality, the coordinate plane cannot be damaged as it is not a tangible thing.{{citation needed}} | {{w|Coordinate planes}} are used in math for drawing graphs. The joke here is that a small section has been "closed for maintenance," likening the concept of a coordinate plane to an actual physical platform used by math, which is therefore vulnerable to damage such as is shown in the comic. In reality, the coordinate plane cannot be damaged as it is not a tangible thing.{{citation needed}} | ||
| Line 22: | Line 22: | ||
Closure can also be used in another sense, relating to the topology of a set; roughly speaking, a description of what parts of the set are "close" to others. In this sense, if one takes the closure of a plane with a hole, the result is indeed an intact plane, provided the hole is sufficiently (infinitesimally) small. | Closure can also be used in another sense, relating to the topology of a set; roughly speaking, a description of what parts of the set are "close" to others. In this sense, if one takes the closure of a plane with a hole, the result is indeed an intact plane, provided the hole is sufficiently (infinitesimally) small. | ||
| − | The title text notes that 3D graphs that cross the relevant x and y coordinates, but with non-zero z coordinates whilst in that zone, should be fine, since the hole only exists in the plane where z = 0. However, if they pass close - i.e. the z coordinate is small in this region - they should be wary of two dimensional graph lines suddenly | + | The title text notes that 3D graphs that cross the relevant x and y coordinates, but with non-zero z coordinates whilst in that zone, should be fine, since the hole only exists in the plane where z = 0. However, if they pass close - i.e. the z coordinate is small in this region - they should be wary of two dimensional graph lines suddenly becoming three-dimensional and interfering with them. This could be because they have intentionally entered three-dimensional space to avoid the closure, or possibly they have inadvertently been 'launched' above/below the plane by the torn and warped edges of the surface. Or they simply fell into the hole, thus entering 3D space. This is similar to warnings to road traffic in open lanes being warned of traffic merging from lanes that have been closed due to works or any other general warning of increased congestion upon a parallel route used as a diversion. |
| + | |||
| + | The concept of 2D objects suddenly entering 3D space — in a way that creates interesting drama and conflict — is the subject of a book, Flatland, that Randall has referenced repeatedly, such as in [[721: Flatland]]. | ||
==Transcript== | ==Transcript== | ||
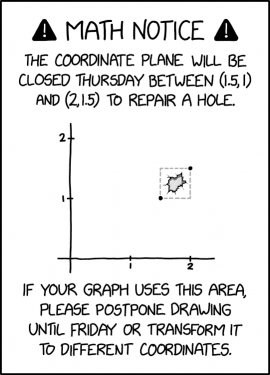
| − | :[A coordinate graph is shown with both | + | :[A coordinate graph is shown with both axes unlabeled but with two labeled ticks. In the middle of the shown area of the graph there is a hole torn in the white "fabric" of the graph's plane. It has jagged edges and lines runs away from the hole in all directions. The area visible through the hole is covered in thin gray lines, and the edges of the hole cast shadows onto the surface below. Two points are marked on the graph at coordinates (X,Y) of (1.5, 1) and (2, 1.5). These two dots marks two of the corners of a square drawn with gray dotted lines, The square completely surrounds the hole. Above the graph there is a very large heading, with black danger triangles with exclamation marks in them, on either side of the heading. Below this there are three lines of text. And below the graph there are four more lines of text.] |
| − | + | :<big>⚠ Math Notice ⚠</big> | |
| − | :<big> | ||
:The coordinate plane will be closed Thursday between (1.5, 1) and (2, 1.5) to repair a hole. | :The coordinate plane will be closed Thursday between (1.5, 1) and (2, 1.5) to repair a hole. | ||
:Labels on Y-axis ticks: 1 2 | :Labels on Y-axis ticks: 1 2 | ||
| Line 37: | Line 38: | ||
[[Category:Charts]] | [[Category:Charts]] | ||
[[Category:Math]] | [[Category:Math]] | ||
| + | [[Category:Flatland]] | ||
Revision as of 14:50, 21 August 2023
Explanation
This comic is a "Math Notice," which is presumably a warning or reminder for mathematicians or others who interact with the field of mathematics, in a similar way to how a "Travel Notice" may prewarn drivers of planned road closures for repairs (or rocketry). It also has similarities to that of a Notice to mariners or air missions, where nautical or aeronautical navigation might be impinged by an area (or volume) that should be kept clear from in the near future, and to notices from websites or software providers about planned maintenance, which alert users about upcoming outages. Specifically, this notice advises those who are using the coordinate plane to avoid drawing any graphs in the area with a hole until the damage is patched or fixed.
The joke may have been inspired as a response to the 2023 China balloon incident, which occurred a few days earlier. This had occasioned one of the largest temporary flight restrictions, with a closed airspace as a response, in U.S. history.
Coordinate planes are used in math for drawing graphs. The joke here is that a small section has been "closed for maintenance," likening the concept of a coordinate plane to an actual physical platform used by math, which is therefore vulnerable to damage such as is shown in the comic. In reality, the coordinate plane cannot be damaged as it is not a tangible thing.[citation needed]
Closure in mathematics can be a term relating to sets, specifically operations on sets, and a coordinate plane is a particular set of numbers. A set is closed under an operation if all the "answers" to the operation are also in the set. The coordinate plane is said to be closed under vector addition for example - adding together any two coordinates produces another coordinate in the plane. Many functions and operators may be said to have closure on the real plane, and this comic may be a pun on that term. However, if there actually is a hole in the plane, then suddenly the plane will no longer exhibit closure.
Also related to closure is the closure problem. Put simply, the closure problem is to find the highest or lowest weight of a closure in certain types of graphs. This comic may also be talking about the closure problem, as it talks about a hole in the graph, and to minimise it would be referring to the closure problem.
Closure can also be used in another sense, relating to the topology of a set; roughly speaking, a description of what parts of the set are "close" to others. In this sense, if one takes the closure of a plane with a hole, the result is indeed an intact plane, provided the hole is sufficiently (infinitesimally) small.
The title text notes that 3D graphs that cross the relevant x and y coordinates, but with non-zero z coordinates whilst in that zone, should be fine, since the hole only exists in the plane where z = 0. However, if they pass close - i.e. the z coordinate is small in this region - they should be wary of two dimensional graph lines suddenly becoming three-dimensional and interfering with them. This could be because they have intentionally entered three-dimensional space to avoid the closure, or possibly they have inadvertently been 'launched' above/below the plane by the torn and warped edges of the surface. Or they simply fell into the hole, thus entering 3D space. This is similar to warnings to road traffic in open lanes being warned of traffic merging from lanes that have been closed due to works or any other general warning of increased congestion upon a parallel route used as a diversion.
The concept of 2D objects suddenly entering 3D space — in a way that creates interesting drama and conflict — is the subject of a book, Flatland, that Randall has referenced repeatedly, such as in 721: Flatland.
Transcript
- [A coordinate graph is shown with both axes unlabeled but with two labeled ticks. In the middle of the shown area of the graph there is a hole torn in the white "fabric" of the graph's plane. It has jagged edges and lines runs away from the hole in all directions. The area visible through the hole is covered in thin gray lines, and the edges of the hole cast shadows onto the surface below. Two points are marked on the graph at coordinates (X,Y) of (1.5, 1) and (2, 1.5). These two dots marks two of the corners of a square drawn with gray dotted lines, The square completely surrounds the hole. Above the graph there is a very large heading, with black danger triangles with exclamation marks in them, on either side of the heading. Below this there are three lines of text. And below the graph there are four more lines of text.]
- ⚠ Math Notice ⚠
- The coordinate plane will be closed Thursday between (1.5, 1) and (2, 1.5) to repair a hole.
- Labels on Y-axis ticks: 1 2
- Labels on X-axis ticks: 1 2
- If your graph uses this area, please postpone drawing until Friday or transform it to different coordinates.
Discussion
Is there significance to the fact that the axes aren't labeled in the warning? Can I plot y = 0.75x today or not?Brossa (talk) 15:05, 8 February 2023 (UTC)
- you cannot because it intersects the given square as shown in this desmos thing i whipped up in 2 seconds: https://www.desmos.com/calculator/zb9nbrl6s5 172.70.43.29 15:38, 8 February 2023 (UTC)Bumpf
- I can if the forbidden coordinates are 1 ≤ x ≤1.5 and 1.5 ≤ y ≤2172.70.131.66 15:56, 8 February 2023 (UTC)
- In the absence of other information, assuming horizontal x and vertical y would be conventional. --141.101.98.145 19:15, 8 February 2023 (UTC)
- I can if the forbidden coordinates are 1 ≤ x ≤1.5 and 1.5 ≤ y ≤2172.70.131.66 15:56, 8 February 2023 (UTC)
"Hole" is also sometimes used to mean a particular coordinate on a function which is discontinous at some point but could have a value (for example sinx/x with a hole at (0,1)). 172.70.206.92 19:18, 8 February 2023 (UTC) Randall listed 2 points, yet the cordoned off area is a square. 2 points define a line, not a square, he really should have thought of that. How is someone to know the invalid points without the diagram? Even with the diagram, we don't know whether points on the boundary are included! Is the line y=1 a valid line to draw? THESE ARE QUESTIONS THAT NEED TO BE ANSWERED RANDALL BE MATHEMATICALLY RIGOROUS NEXT TIME.
- Right! A hole pops up in rational functions when there's a term that appears in the numerator AND the denominator. However, it does not mean the graph is broken; just that there is no defined y-value at the x-value of the hole. ----Theunlucky (talk) 16:55, 9 February 2023 (UTC)
- One reason could simply be the alignment between the coordinates and time. Reading out the numbers without paying attention to the mathematical punctuation you can form the sentence "the coordinate plane will be closed Thursday between 1:51 and 2:15 to repair a hole", following the typical structure of such a notice to not just provide a day but a time.
Ironically, the notice makes it sound like using y=1 is fine, and the affected region is only strictly greater than y=1. That would make the region that's closed an open set, and the region that's open a closed set. 172.70.110.230 22:46, 8 February 2023 (UTC)
- Right! A hole pops up in rational functions when there's a term that appears in the numerator AND the denominator. However, it does not mean the graph is broken; just that there is no defined y-value at the x-value of the hole.
🚧 DETOUR 🠕 KEEP WITHIN MINKOWSKI CONES ⛔ DO NOT ENTER Y < |X| 🚧 162.158.90.38 23:37, 8 February 2023 (UTC)
So the joke is that the coordinate plane is closed when there's damage that causes it not to be closed? Barmar (talk) 23:44, 8 February 2023 (UTC)
Aw man, I was really looking... forward... to doing math today. 172.71.222.76 11:58, 9 February 2023 (UTC)
I thought the title text was referring to the danger of lines on a 2d graph "falling through" the hole and inadvertently gaining a third dimension, which might collide with graphs at z=-1 etc. 162.158.34.75 16:14, 9 February 2023 (UTC)
My RSS reader picked this comic up at exactly midnight UTC on Feb 8, which stood out to me because usually they seem to be posted later in the day. Danielp82 (talk) 04:02, 10 February 2023 (UTC)
This comic reminded me of Complex Analysis, where we integrate in circles around singularities of complex functions (aka holes). See Cauchy integral formula. Maybe we should mention that in the explanation. 172.71.154.39 07:29, 10 February 2023 (UTC)
- The beauty of the Wiki is that you can add it yourself, if you think you can word it relevently. Or anyone who now wants to. (Whoever does, note that
{{w|Cauchy's integral formula}}, or an altered text version like your{{w|Cauchy's integral formula|Cauchy integral formula}}, would be the prefered wikilink format to use.) 141.101.98.151 08:08, 10 February 2023 (UTC)
I made the unfortunate but defensible change from "airmen" to "air missions". The FAA re-consecrated "NOTAM" to the gender-neutral (and execrable) form on 2 Dec 2022. The "airmen" form may still be in use by ICAO or nations other than the US. Der57 (talk) 10:52, 10 February 2023 (UTC)
Don't you think it's uncharacteristic of Randall to deviate from the normal math practice of placing the x coordinate first before the y coordinate when not explicitly identifying them? Furthermore, each coordinate is backwards from the convention of smaller number first, then larger? This is so out of step for him I think he did it deliberately and we're missing a subtle joke... Paso Dan (talk) 16:38, 10 February 2023 (UTC)
- What are you talking about. He lists the number as (X, Y) completely normal with X on the lower axis and Y on the one going up and so does the numbers he gives follow normal style. Also he starts with the number that comes first on the x-axis. See no reason to start with another, and this is also relevant for making it look like he is given a time period. Seems to me you must have made a mental mistake when you wrote this? --Kynde (talk) 17:35, 10 February 2023 (UTC)
- Yeah, like Kynde said, he's using standard notation... the region (1.5, 1), x = 1.5 and y = 1, and (2, 1.5), x = 2 and y = 1.5... X first, then Y, standard. He even plots those points, with dotted lines denoting a square "cordoned" off... And when sorted, graph points usually go left-to-right (so, ascending order by X), which he did. NiceGuy1 (talk) 05:42, 11 February 2023 (UTC)
- I get what Paso Dan is saying, and it was my impression as well. Indicating that the coordinate plane is closed between two points (A,B) and (C,D) doesn't by itself tell you whether the closure is along the line between those two points, or a circle with a diameter running from (A,B) to (C,D), or some other 2-D shape. The diagram indicates a square with corners at (A,B) and (C,D) and sides parallel to the axes, but that information isn't in the text. If on the other hand it's interpreted as a closure for the region where x is between 1.5 and 2 and y is between 1 and 1.5, you get a full description of the closed area. --Brossa (talk) 17:36, 18 February 2023 (UTC)
- Two points are capable of defining an axis-aligned rectilinear quadrilateral, or a diamondoid (if you decide to use the convention of a 45-degree skew), or an arbitrarily-rotated square (defining one of the long diagonals). Pretty much any other quadrilateral (or other shape) needs further pre-agreed presumptions or more points of definition. A circle can be defined by three (non-colinear, non-identical) edge-points, or an oval (even skewed) by, at the very least, a third value/coordinate in different manners.
- (Oh, ok, you could assume a circle defined by just two points (diametrically opposed), the circumscircle to the arbitrary square, above, or even the circle that is inscribed to it. Or "centre and point on radius". From that stage you could indicate further polygon that can be circumscribed thusly, oriented either constantly to the axes or to the direction defined by the (initial) radial point. So your toolkit can feature a "drawShape(coord1,coord2)" for all kinds of areas, one for each possible kind and treatment.)
- Yet, in the absence of any detail other than "this defines an area", two surface coordinates almost certainly will be best assumed to defibe a rectangle (or a graticule, given latitude/longitude coords) and two 3D coords ones a cuboid/whatever. 172.69.79.185 20:27, 18 February 2023 (UTC)
Finally, I get an explanation why I've seen so many mentions of "China balloon" lately (and the picture of the moon with a silhouette on it, like the one I saw with X-Wings photoshopped flying off to it, didn't even realize they were related). :) I didn't feel like Googling it, figuring it would come to my attention eventually. LOL! NiceGuy1 (talk) 05:35, 11 February 2023 (UTC)