2625: Field Topology
| Field Topology |
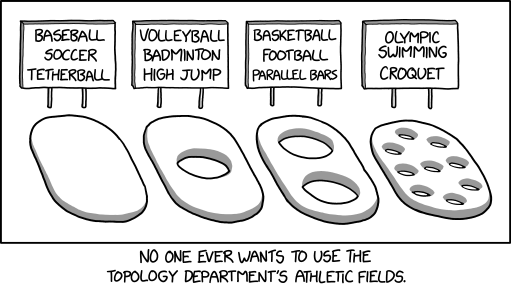 Title text: The combination croquet set/10-lane pool can also be used for some varieties of foosball and Skee-Ball. |
Explanation[edit]
Field Topology is a subject in mathematics, but in this comic, Randall is instead examining the topology of playing fields used for various sports. The comic strip depicts a situation in which the common practice of multi-use athletic facilities has been organized by the "topology department" and constructed to be shared by all sports whose normal playing fields are topologically equivalent. One key assumption in topology is that you can ignore the specificities of shape, size and material of the objects concerned. This presents an amusing contrast as the "equivalent" topology department playing fields are actually not very appropriate for the activities listed in the comic, as the standard positioning, size and shape of hoops, nets and bars and the material of the field itself are not equivalent to the real playing fields used for those activities.
(Not to be confused with mathematical fields, or the Fields Medal prize -- although the concept is likely a further pun in the comic, as math (including topology), and most things once can imagine really, are mostly performed ("played") within mathematical fields.)
In topology, shapes which can be smoothly deformed into one another without adding or removing holes are considered equivalent. A topological hole is an area of the nominal space (or area, or other manifold) through which nothing restricted to this topology can pass. A loop is a path across the allowable territory of a topology (or a viable circuit to make through the world it describes) that end up where it started. For example, when describing the space taken up by a solid object such as a coffee mug, the handle forms a loop with a hole through it. If a loop cannot be tightened (ultimately adjusted to take a shorter path) down to a single point, then it must be wrapped around at least one "topological hole", and you have separately unique paths (or points, i.e., on different disconnected topologies) where you cannot adjust one loop to take the route of another without severing a looped path and reconnecting it.
When describing a negative space, such as the space around an archway, the 'hole' would be the material of the arch itself. This is because a loop formed by a ring around any part of the arch material can only be shortened to a finite length, not to a point; the 'hole' is the arch-shaped obstruction which forces the existence of these loops. A basketball hoop connected to the ground forms a similar obstruction with a loop through it, so the space around the hoop contains an equivalent hole. In this comic the topology department has analysed the spaces where various sports are played by the number of such obstructions in the playing area. Each space depicted in the comic is then signposted with the sports which are played on a field with that number of holes.
Baseball, tetherball and soccer are played on fields which are continuous in three-dimensional space. This means it is possible to traverse any path around or over any of the structures defining the field, while there are no obstructions which can be traversed through in a loop around them. The goals on a soccer field presumably do not create holes because the goalposts and crossbar are connected to the field by the net; Randall apparently considers these to form continuous surfaces which do not allow loops through them.
Volleyball and badminton are played using a net suspended from poles, and the high jump has a bar that contestants jump over. The structure formed by the net or bar and the supporting poles can be considered to be a "hole" through the playing field, as a path over and under the net/bar forming a loop cannot be contracted to a single point, so their playing fields in the comic all have one "hole".
A basketball court has two hoops. Parallel bars can be thought of as two archways. Both have opportunities to pass through either (or both) structures, and so the material of the structures define a hole in the topological abstract of the playing 'space'. Since we are told that these sports fields belong to the Topology Department - and are not necessarily generalized to all sports fields - we might assume that their "football" field is either for rugby or for American football using H-shaped uprights.
An Olympic-sized swimming pool has ten lanes, and thus nine lane dividers which are fastened to the walls of the pool at each end, creating topological holes through the play area. Each hoop in croquet is similarly a hole through the space; while most versions of croquet use six hoops, nine hoops are used for "backyard croquet" which is played recreationally in the United States and Canada. The fact that the space in a swimming pool is typically filled with water[citation needed] has been overlooked by the topology department.
As mentioned in the title text, this last configuration is also homeomorphic to a foosball table (with each rod sustaining the player figures above the table defining a hole) or a Skee-Ball lane (which is even more straightforward, as it is just a plane with several holes in which to throw balls). These "fields" don't actually have the same number of holes, but are apparently lumped together by the Topology Department as having "many" holes.
Unfortunately, the Topology Department does not seem to have a field for hurdling events.
Transcript[edit]
- [A row of four signs, each held up by two posts, followed by a row of four rounded lozenge shapes, one for each sign. The signs and lozenge shapes are shaded as if three-dimensional objects, all being flattish with a small third dimension; the four lozenge shapes each have one pair of sides horizontal and the other pair at a slight angle from vertical, denoting a horizontal plane perpendicular to the signs extending "out" towards the viewer, which places each shape "in front" of its sign. All but the first lozenge shape have various numbers of ellipses within the shape - ovoids shaded to denote holes piercing through the objects.]
- [Leftmost sign:]
- Baseball
- Soccer
- Tetherball
- [The shape below this sign contains no ellipses.]
- [Second sign from left:]
- Volleyball
- Badminton
- High jump
- [This shape has one large ellipse in the center.]
- [Third sign:]
- Basketball
- Football
- Parallel bars
- [This shape has two large ellipses - one in the top half and one in the bottom half.]
- [Fourth and rightmost sign:]
- Olympic swimming
- Croquet
- [This shape has nine small ellipses - eight arranged symmetrically towards the edges of the shape and one in the center.]
- [Caption underneath the signs and shapes:]
- No one ever wants to use the topology department's athletic fields.
Discussion
First 172.70.86.64 12:50, 27 May 2022 (UTC)
To me the topological fields look like toilet seats with three more or less seashells. --Gunterkoenigsmann (talk) 16:19, 29 May 2022 (UTC) Why is football on the two-hole field? Where are the holes? I don't think the goal posts in American football introduce any since they're not closed. Maybe it's soccer? 172.69.68.88 12:58, 27 May 2022 (UTC)
- I think it is because the goal posts extend into infinity and the topological definition of a hole: something you can draw a circle around that you cannot contract to a point. [the user placed a horizontal rule instead of a signature by accident.]
- Well, you might still be able to call them holes. They would be if they were fully rectangles. --BlackBeret (talk) 12:59, 27 May 2022 (UTC)
- Gridiron football's field contains two areas (the endzones) that can be thought of as not being part of the "normal" field of play, for lack of a better way of saying that pre-coffee. Association football likewise has the areas within the nets. Noëlle (talk) 13:05, 27 May 2022 (UTC)
- My immediate thoughts were also that football (soccer) and football (gridiron) are the same, or indeed the other way round. In both cases the closed hole (assuming not a Y-like vertical holder, but H-like as per rugby football) plays no more or less topological part. Threading through the hole from behind has no relevence in either, and in fact defining it as a region that is 'a special enclosed gap with meaning' (which doesn't really matter in the topology sense, just like golf would be a topologically hole-less surface and as a coffee-cup's inside 'dimple' doesn't count, just its handle-hole that makes it equivalent to a doughnut) actually counts for something in association football. 172.70.162.155 13:32, 27 May 2022 (UTC)
- It's not the space bounded by the goal that is the 'hole' - it's the goal post itself (or in the case of the high jump, it's the bar, not the space under it). The reason soccer doesn't have 'holes' where the goals are is that they're positioned on the edge of the playable area - you can't play around the bars, because as soon as you cross the goal line you're out of play. And it doesn't matter whether it's a Y-shaped or H-shaped goal - topologically, they both form one continuous 'hole'. 172.70.91.80 13:37, 27 May 2022 (UTC)
- I don't think that's the reason why soccer doesn't have holes. The goalposts in football are also outside the playable area, and so are the poles in volleyball. I think soccer is listed as zero-holes because soccer goals are typically not fixed to the field, and are instead separate objects that can be dragged around and removed from the field. On the other hand, the same is true of volleyball and badminton nets (and those nets contain many holes!) so the comic seems a bit inconsistent.172.70.175.146 14:05, 27 May 2022 (UTC)
- Speaking from a "football is soccer" nation (well, mostly, the exceptional subregions would argue that it's rugby) a soccer goal is typically not draggable around the field, but permanent (or a unit frame that has to be painstakingly hoisted out of the ground if you don't want them in your football stadium, when you repurpose it for other purposes) and it's only the optional net that gets added to the park's permanent goalposts for the official five-aside competition evening or day of the weekend. Draggable goalposts need a further level of intermediate organisation that goes beyond the typical "shipping container with windows cut in it (with shutters bolted over them) as a cheap changing room/officials' cabin" that might be found near the edge of the field but rarely even has as much as a corner flag left in them, between games".
- I presume that US 'football' posts are considered holes because they are an infinitely-tall window (even though the delineating poles only reach so high) that is a meaningful slice (where the goal is, you have to loop around it in mutually different unsimplifiable paths to reach the other side), but then that should make for two holes per end, if you count getting a field-goal and then returning round the sides (or vice-versa) as another valid surface-path.
- ...but, yeah, I can imagine the problem of definition (and cultural famiarity) here is going to produce more problems even than the understanding of topology. One of the less internationally-accepted comics, this. 172.70.85.177 18:51, 27 May 2022 (UTC)
- O_O . Randall is united-statesian, so football means the thing where you tackle each other and hold the ball in your hands. I've never been into football, and I've always seen it with two large goal posts with a horizontal bar between them. The hole is formed under the horizontal bar. When I played football in computer games, you had to get the ball over the horizontal bar. After this, I'll search the web to see if the horizontal bar still exists. Regarding soccer, there aren't two holes because the nets are closed at the back. You cannot pass through the field structure by going through a goal: you bump into the net the ball bounces off of when a goal is made. So, Randall is considering soccer fields topologically equivalent to a plane (ignoring all the holes in the netting). 172.70.114.229 14:58, 28 May 2022 (UTC)
- I looked up the goal thing and found that what I was imagining are called H-frame or H-style goal posts. Not the norm; the have two posts instead of one. I'm a weirdo that I thought they were what was up. But Randall could have been thinking of H-frame goals. 172.70.230.63 15:04, 28 May 2022 (UTC)
- Many high school and amateur football fields still use H-frame goals. The resulting space can be used as a goal in some other sports. That does raise the question of why they didn't just have one field with lots of holes, and just plug the ones up that aren't needed for the sport being played. 172.70.134.191 15:57, 28 May 2022 (UTC)
- H-frames are indeed the norm for American football in the everyday world. Only the professional and highest tier colleges use the more expensive Y-frames (which have the advantage of being harder for players to run into them). In fact, it is very common for the lower space of the H-frame to have the dimensions of a soccer goal, so that the field can be used for both association and gridiron football. If some Americans get go to the nearest park to play football, or go out on a weekend to see a local team (i.e. high school or community college) play, they are likely using H-frame goals. These are not the sorts of games that are televised, but there are thousands of such games played for every one on a fancy field with a Y-shaped goal. 172.69.68.31 13:02, 31 May 2022 (UTC)
Tetherball, in many variants, does contain an obstruction -- the pole, which you're not allowed to touch. The Topology Department is getting tired of having to switch out the fields. Noëlle (talk) 13:05, 27 May 2022 (UTC)
- But you can surely jump over it, so it's topologically the same as a zero-height pole... 172.70.162.155 13:32, 27 May 2022 (UTC)
- Tetherball does not have a *hole*. The pole, rope, and ball are just a stretched out bit of the continuous surface.
Croquet has six hoops and a peg. How does that make for nine holes? Is it including the opponents' two balls as holes? And if so, why aren't opposing players counted as holes in the other sports? 172.70.91.80 13:26, 27 May 2022 (UTC)
- [1] 'Nine-wicket croquet, sometimes called "backyard croquet", is played mainly in Canada and the United States, and is the game most recreational players in those countries call simply "croquet".' (Wikipedia) 172.70.126.215 18:58, 27 May 2022 (UTC)
American football goals are Y-shaped. Rugby goals are H-shaped. Did... did Randall get those confused? Also, I fail to see how basketball and American football get two, croquet gets a bunch, but soccer gets zero. Aren't soccer goals (in-game at least) basically the same shape as croquet wickets, just waaaay bigger? Granted, I don't know anything about topology and I came to this wiki specifically cuz I'm dumb, so I'd love if someone could splain this all for me ;) --mezimm 172.69.69.170 13:37, 27 May 2022 (UTC)
- The soccer goal has a net, so the ball can't go through it. Topologically it's just a wall (Randall seems to be ignoring all the tiny holes in netting, presumaby because they're smaller than the balls so they're insignificant to the sports). Barmar (talk) 14:10, 27 May 2022 (UTC)
- I agree with that explanation - the net is the only thing that makes the soccer field not to have holes. It should be included in the comic explanation.
- The hole for the volleyball only makes sense taking in account that the bottom of the net doesn't reach the floor, although this space is not used in the game.--Pere prlpz (talk) 14:18, 27 May 2022 (UTC)
- I agree about soccer; the explanation should be that soccer goals (with net) are topologically part of the plane. The same is true of ice hockey, even though you can travel "around" the net, it is topologically part of the field with no holes. As for (American) football, the topology only makes sense for H-shaped goals, which are more often seen on primary/secondary play fields than in higher level play. Aramisuvla (talk) 16:03, 27 May 2022 (UTC)
- Agreed. Soccer goals are shaped such that their bottoms connect smoothly to the ground in a single continuous piece. So they are topologically equivalent to the plane. This wouldn't be the case if not for the back part holding the net. That's unlike basketball hoops, which are actual holes. The holes in football must be referring to the H-shaped uprights that were standard until 1967 in professional leagues and are still seen in some high school fields and even a couple college fields. 172.70.131.128 03:08, 28 May 2022 (UTC)
- EDIT: I should point out that the net actually has, like, hundreds of holes. But I think the net here is being treated as a continuous sheet. 172.70.126.215 03:10, 28 May 2022 (UTC)
The group link pointing to group (mathematics) doesn't bear any relation with the sentence or the comic. I would remove the link.--Pere prlpz (talk) 14:18, 27 May 2022 (UTC)
- The joke seems important to me because their no consideration of the word 'field' being a math pun, and it raises the idea in readers. 162.158.79.74 15:11, 28 May 2022 (UTC)
- When I first saw the comic title I assumed that part of the joke would be a pun on the word "field" being used for both sports and math. And even though the comic doesn't explicitly make this joke, I'll bet it inspired Randall. It's worth mentioning. Barmar (talk) 16:43, 28 May 2022 (UTC)
In rugby (both League and Union) the goalposts are within the field of play: significant game activity takes place behind them. This is not the case with soccer. I have no clue what difference this makes topologically. [[Special:Contributions/|]] ([[User talk:|talk]]) (please sign your comments with ~~~~)
I've been thinking about this classification system, and can't quite work out the baseline for it. I think we're supposed to assume that the whole 3d manifold is represented in a 2d 'field', or at least any path through the air flattened to an arbitrarily thin surface 'bulge' during topological rationalisation. But there are several possible field-of-play definitions we can be using...
- A single valid 'play' or traversal
- For ball-sports (or indeed other play-objects) this could be where the item can travel. But in this case I think almost 'all' codes of football are Type 1 (first of the topologies) as almost every football code deals with both 'goal' and 'endzone' (where valid) as the same as a hole (dimple) in golf... It goes into it and it might as well come out of it again, there's no continuation of play 'through the defined' space, and so the topological hole (the barrier defined the scoring membrane's edge) never comes into play.
Unlike in Gridiron, where a touchdown doesn't even need the 'ball' to touch the ground, rugby (league and/or union, and possibly further derivatives) requires this and a player can fail to score a Try if (s)he passes bodily over the line but is unable to plant the ball (not allowed to throw/drop it) and I'd have to check what happens if the defending player(s) keeping them sufficiently off the ground (assuming that's done in an allowable fashion) returns the intended scorer back over the line via a circuitous route around /back-through the suspended goal-mouth (above the cross-bar, between the verticals)... They keep changing those kinds of technical rules, so I can't be sure of the current technicalities involved.
Likewise, a volleyball or shuttlecock that passes under the net-top-edge is out of play, so it is really a Type 1 under this definition. (Might as well be a solid barrier, floor-to-top-height, rather than a thin bar or a partial net.)
The basketball case is interesting. Although a dunk ends the play of the ball, I'm not sure if the path of a ball up through the hoop does not. In that circumstance I could believe it is a Type 3 case, but if that's a game-stopping thing then Type 1.
Croquet is indeed a varying number of paths through (I think) an unordered set of holes, or at least nothing to say that they can be taken out of order (or 'un-passed-through'), and you can't necessarily restrict a 'play' to one shot at a time if certain conditions allow you to play on, so dodging in and around all scoring zones defined by the hoops gives you something like. - For player/competitor/participant movement, similarly passing under the bar is not valid for the High Jump.
I don't think there's anything to stop such transitions upon the Parallel Bars, but it is much more a feature of the Uneven Parallel Bars, whereas from what I've seen of the sport, the even-variety tends to be topologically used much as the pommel-horse.
Players of football (American variations certainly, rugby of course, proper football if you don't bother with the nets) are not restricted from passing through the scoring area (either way) on a circuitous path that may be off the field of play but isn't off the field of players.
For the Olympic Swimming, I'm not suring porpoising over and under the lane-delineations is a thing, so I would have said that (under this definition), it should be a number of entirely disconnected Type 1 'zones', with no valid movement between them at all.
So far as I'm aware, there are no rules for/against croquet players passing through hoops (intentionally or perhaps because they severely annoyed an opponent) so maybe that stands in this case, too. Ditto for basketball, if hoisted. Although in both cases it may prevent the balls passing through immediately afterwards, without game-stoppage to resolve the issue.
- For ball-sports (or indeed other play-objects) this could be where the item can travel. But in this case I think almost 'all' codes of football are Type 1 (first of the topologies) as almost every football code deals with both 'goal' and 'endzone' (where valid) as the same as a hole (dimple) in golf... It goes into it and it might as well come out of it again, there's no continuation of play 'through the defined' space, and so the topological hole (the barrier defined the scoring membrane's edge) never comes into play.
- If it's a game's-worth of play, then the status of the basket in basketball (unlike the pocket in snooker/pool/some-versions-of-billards) might be defined by the topological-hole-that-is-the-physical-hole's-edge, rather than treat it as the old basket-with-bottom from which the precusor to the net-ring almost immediately evolved. And the same could be said about the suspended scoring-hole (whether supported as Y-post or an H-post, the lower limb(s) are merely physical necessities that play little part in the gameplay specifics except as a general hazard to avoid, it is the crossbar and verticals-to-infinity (and the infinity itself) that is the gap through which a circular path cannot be rationalised back to a point). For most of the rest (including the participant-paths, with there being nothing to stop the traversal of a footballer of whatever stripe jumping the cross-bar, but that may only mean something in the topology of some variations, as far as the game is concerned...) it seems meaningless. Even in an Aussie Rules field with four 'posts' per end, and probably more interest in whether jumping onto an opposing player is against the rules or indeed an entirely legitimate and expected tactic.
- The general arena-wide area is a further superset (perhaps with no additional complications, i.e. exactly congruent) of the field-of-play(er) definition. For coin-operated table-top games (foosball/table-football) the path from each goal may (additionally to any on-top topological loop-disconnections) force passage of the ball underneath and out into the new-play insertion spot. So add a couple more (unidirectional) paths, at least. Or six for a coin-operated pool/etc table, and I assume the Skeeball (not something I'm familar with, at least by that name) is defined that way already...
Sorry, I found I needed to say a lot more than I thought I did, so the first point (and sub-points) went on a bit and I cut down what I might have said for the following points. I may come back to re-edit this. I've got a handy little table, in mind, but I'm not sure it'll work much better to summarise everything I've been cogitating about for most of today while away from the keyboard... 172.70.162.5 15:57, 28 May 2022 (UTC)
- The "hole" in the goalpost in American football is relevant for field goals, not touchdowns. Barmar (talk) 16:43, 28 May 2022 (UTC)
- I'm not sure it's relevent for either. The field-goal passes over the crossbar and between (but also maybe above) the raised verticles, but that route is topologically the same as one above the crossbar but wide, which is in turn the same as one rolling along the ground and wide... Or indeed carried across just like most touchdowns (any that isn't run through the middle of the H-post', un-netted but otherwise soccer-like 'goalmouth' lower section).
- Possibly running around the post(s) that support the field-goal defining beams counts as the path around the topological hole because any change to that route that attempts to transform it to a useless loop within the main field of play must either (at some point) pass through the support for the crossbar or else wholly through the region that defines (in one direction, at least) the goal-scoring area. Can anyone get Word Of God in his intentions, here? It looks weird, to me. 172.70.162.77 03:48, 29 May 2022 (UTC)
As has been alluded to, this must be an American university's topology department. A rest-of-the-world university would include four holes for cricket. 172.70.134.191 17:48, 28 May 2022 (UTC)
Ok, this is my (not yet properly tabularised, or properly wikimedialinked) idea of all the kinds of information I'd suggest go in there.
But it's a monstrocity and I don't want to remove the very useful existing information already in the Explanation (that may even be better/more accurate than my interpretation).
...so here it is for review. If anything in it is useful to anybody else as inspiration for future edits then... well, your choice!
- Click to expand:
Competition |- Field diagram |- Usage description |- Topology |- Type 1 Field |- (First image in comic.) |- Any path looping around this area can be moved at will and shrunk to just one point that could result from any other path. |- A homogonously flat lozange surface with no other notable features. Baseball |- (Partial!) https://en.wikipedia.org/wiki/Baseball#/media/File:Baseball_diamond.svg |- The playing area for baseball contains many important physical features for scoring and playing purposes, but is essentially one flat area (and continuous airspace) when you disregard the elevation of the pitcher's mound or even the outfield fence and stands (for any ball that carries that far, upon being hit). |- Randall explicitly classes this in the Type 1 diagram, and there isn't any obvious reason to argue this point. Association Football ("Soccer'"/"Football") |- https://en.wikipedia.org/wiki/File:Football_pitch_metric_and_imperial.svg |- An unobstruted rectangular playing area with a goal formed of two vertical posts connected between the tops by a crossbar. In official competition (and where otherwise desired) there is a net stretched behind each goalmouth to stop any ball that passes completely through it (with or without hitting any of the posts), although games can be played with no net in place, or in street/schoolyard situations by goals defined only as a goalpost-like markings painted upon a solid wall (hitting the wall within the bounds of the painted line constitutes a goal, give or take arguments about whether it counts if it hit the line). |- Stated by Randall as a Type 1 (a single unobstructed zone), which is likely due to the 'pocket' of the net-backed goalmouth being nothing more than a straight extension of the playing area. However, an un-netted set of goalposts might be considered a Type 3, with each set of goalposts defining an impassible frame (the hole in the topology, not the same thing as the physical hole formed by the goal-frame) within which the balls can freely pass and return not through the goalmouth, or vice-versa. Tetherball |- https://en.wikipedia.org/wiki/File:Tetherball_in_Georgetown,_Seattle,_Washington.jpg |- A ball attached to a cord anchored at the tip of a pole that is in turn stuck in the ground. |- Although the mechanism used to allow free swivelling of the tether around the pole may be quite complex (including being looped around a helical thread to help register how many excess orbits of the pole the ball has made in either direction), the basic premise can be simplified to a single extrusion from the playing area, which is topologically identical to a playing area with no extrusion at all. Thus Randall properly states this as a Type 1 variant. Type 2 Field |- (Second image in comic.) |- Any path that canot be shrunk to just one point will be pass around the unpassable hole in the topology. |- A homogonously flat lozange surface with a single central hole in it. Volleyball |- https://en.wikipedia.org/wiki/Volleyball#/media/File:VolleyballCourt.svg |- A volleyball court consists of a flat area disected by a raised net in the centre. Valid shots pass over the net, but it is possible for the ball (or players) to pass between the net and the floor. |- Randall lists this under the Type 2 diagram. An argument can be made that the net could effectively reach to the ground, or questions asked about anchoring the net top/bottom to the posts at either side with separate straps (adding left and right 'passages' between the elements of the obstacle that is the net) but he clearly intends the loop around the hole to represent the ability to passing over the net one way and under the net the other (or vice-versa) as a topologically irreducible loop. Badminton |- https://en.wikipedia.org/wiki/File:Badminton_court_3d.svg (Note that this diagram completely abstracts the under-net area away.) |- The net setup is very similar to volleyball, i.e. raised above the ground, with very similar rules regarding valid shots between the areas on each side. |- As with Volleyball, Randall feels justified in this being classed as a Class 2, having similar reasons for this as well as possible arguments against. (Note that another form of Badminton is arguably far more topologically complex!) High Jump |- https://en.wikipedia.org/wiki/File:1912_Platt_Adams5.JPG |- A bar supported at height between two supports. The idea is to successfully pass over the bar (without knocking it off, the bar being only supported to the supports, not firmly attached to them), although a competitor who decides to abort their attempt mid-run might well choose to pass underneath to default the attempt with the least physical and organisational aftermath. |- With an 'above' and 'below' path to potentially loop around (though not in a single jump), Randall chooses to ascribe this as a Type 2. If a competitor displaces the bar, during a failed jump, it can morph the topology into a Type 1 scenario Type 3 Field |- (Third image in comic.) |- Any path that canot be shrunk to just one point will pass around one ot other or both of the holes in the topology. |- A homogonously flat lozange surface with two holes in it, towards each end. Basketball |- https://en.wikipedia.org/wiki/File:Basketball_terms.png |- Played upon a court, at each end of which is a tall pole (or supporting wall or other structure) from which a 'basket' is projected over the playing area. The earliest baskets were an actual closed-bottom basket, but this required climbing up to retrieve balls successfully landed within them. By removing the bottoms of the baskets and, later, using just a hoop (with or without a bottomless net). Points are scored by sending the ball through the basket-loop from above, to be retrieved for further play as it exits below. |- Topologically, the edge of each loop is directly connected to the ground, so it can be smplified as a two-hole Type 3 field (the hole in the field is the impassible rim in the basket-loop). This does not preserve the orientation (or intended unidirectional nature) of the basketball-shot, but this is Topology's fault, not Randall's! American/Canadian Football ("Gridiron"/"Football") |- https://en.wikipedia.org/wiki/American_football_field#/media/File:AmFBfield.svg https://en.wikipedia.org/wiki/File:Canadian_football_field.png |- A unobstructed rectangular playing area and two 'Endzones' at each end. Goalposts are either of an "H" shape or essentially a "Y" (crossbar, upper verticals and a single utilitarian post, usually set back beyond normal playing area with an extension over to hold the crossbar directly over the goal-line. The verticals are tall but are also conceptually projected upwards without limit, for scoring purposes, should a field-goal/etc be kicked high enough to exceed the structures. |- Stated by Randall as a Type 3 (a topological hole at each end of the field), which may represent the bound surrounding the elevated goal-scoring area. Alternately it represents the physical structure of the H-shaped posts which rationalise down to the open-backed ground-touching goalpost footings and the crossbar. Parallel Bars or perhaps Uneven Parallel Bars |- PB: https://en.wikipedia.org/wiki/File:AlejandroonParallelBars.jpg UPB: https://en.wikipedia.org/wiki/File:Paksaltoliukin.jpg |- The Parallel Bars are two horizontal bars supported at roughly hand-height, upon which a gymnast will perform various hand-supported feats strength and coordination. The participant will not usually fully use the space beneath either bar (and between the two supports for the bar), but a will needs the opportunity to grip fully around the bar, especially when the other hand is released for a complicated body movement and it would be impractical or a different discipline entirely to used a 'filled' bar-support. The Uneven Parallel Bars are two similarly supported bars but at two different (and greater) heights, with the performance being generally that of keeping the grip of both hands (or knees/etc) on either one or other of the bars whilst rotating around its axis, when not actively transfering across between the bars themselves. |- Effectively two loops (as per basketball hoops but in a different orientation and scale). The Type 3 topology suggested by Randall is more meaningful for the use of Uneven Parallel Bars, but is probably applicable to the 'even' version in its own way. Type 4 Field |- (Fourth image in comic.) |- Any path that canot be shrunk to just one point will pass around at least one (and possibly several) of the nine holes in this topology. |- A homogonously flat lozange surface with nine small holes dotted into it. Olympic Swimming |- https://en.wikipedia.org/wiki/File:Swimming_pool_50m_2008.svg |- In competitive swimming, a swimming pool is often delineated into lanes (for Olympic purposes, Lane 0 to Lane 9, though usually not all will be used) by floating barriers and other markings. These provide a limited amount of wave-reduction but mostly keep competitors from inadvertently drifting across or into each others' paths. |- Randall considers this setup to require nine 'holes' in the competition area, presumably where the floats pass along the surface of the water, to make a Type 4 field of competition. He must then consider it perfectly possible for competitors to pass under or over these barriers, at will, with complete disregard for the usual competition (and risking disqualification). Otherwise, it might be best considered as (up to) ten separate Type 1 arenas, with just one swimmer in each. Croquet |- https://en.wikipedia.org/wiki/File:Modern_croquet_equipment.JPG |- A game in which a number of metal hoops are placed in the ground such that a given number of players (or teams of players) must each propel their own ball(s), and possibly those of their opponents, through each loop either directly with their own mallet or through contact between balls. Many variations exist with differing numbers of hoops and variations of rules and winning conditions. Randall appears to favour the "Nine-wicket Croquet" popular to North America. |- The topological simplification of nine hoops across a flat surface can be thought of as the Type 4 topology displayed. Table Football ("Foosball"/"Table Soccer") - as per title-text |- https://en.wikipedia.org/wiki/File:Foosball_garlando_aerial.jpg |- An enclosed playing surface with (typically) eight rotatable and extendable bars supporting representative (soccer) 'footballer' figures, ready to strike a small ball across the surface, as might be desired by the two or more opposing players who are each able to control the movements of half of the 'bars' (each team's-worth having a goalkeeper, defence, midfield and attacking 'layer'). By skill and/or luck, the aim is to propel the ball into the opposing's player's goal. On coin-operated games, often the playing area is usually sealed off from direct manual interference, and a ball that goes into the goalmouth finds itself in a lower chamber that stores the ball(s) and deposits them via some feed to carry the ball back up and 'thrown in' towards the centre of the table to start the next attempt at goal. |- With eight bars across, and potentially two goalmouth sinks, this may not actually add up to a nine-hole Type 4 field of play. But presumably Randall is thinking of a version that does. Skee-Ball |- https://en.wikipedia.org/wiki/File:Skee_Ball.JPG |- An arcade game in which a ball is propelled by the player to land in (according to skill) one of various holes in a target-ridden surface (to return back to the player for another go). |- It would depend upon the exact confuguration of Skee-Ball machine but, again, Randall seems to think this matches the Type 4 topology. Further (football) examples, unmentioned
Australian Rules Football ('Aussie Rules'
|-
https://en.wikipedia.org/wiki/File:Footygroundfix.svg
|-
An unobstructed oval field with four simple vertical posts upon the perimiter arcs at each end.
The ball passing between the (taller) central pair of each end's posts (projected upwards indefinitely) is a Goal. Passing between the outer posts and the adjacent central one (or bouncing off these) is a Behind.
|-
Type 1 if the protruding poles are rationalised to zero, without respect to scoring zones. Four or perhaps six topological holes (two or three per end) if respecting the imaginary projections indefinitely upwards for scoring purposes, depending upon if you care about chirality of the ball path.
Gaelic football ('Gaelic') - fields also used for Hurling
|-
https://en.wikipedia.org/wiki/Gaelic_football#/media/File:Gaelic_football_pitch_diagram.svg
|-
An unobstructed rectangular field with an H-shaped set of goalposts at each end, the area below the crossbar often being netted, while the upper verticals being nominally considered as projecting upwards without limit.
Valid balls sent over the crossbar and between the verticals are awarded Points; those sent into the netted goalmouth are Goals (equivalent to three Points for scoring purposes).
There is no in-play use of the area behind the line of the goalposts, unlike various other football codes with similar-looking posts.
|- Topologically, probably considered a Type 1. Goal-shots are into a 'pocket' extension (if nets are used), and Point-shots are topologically indistinguishable from passing over any other part of the boundary line.
Rugby League/Union ('Rugby'/'Rugby Football'/'Football')
|-
https://en.wikipedia.org/wiki/Rugby_union#/media/File:RugbyPitchMetricDetailed.svg
https://en.wikipedia.org/wiki/Rugby_league_playing_field#/media/File:NRL_Rugby_League_field.svg
|-
An unobstructed rectangular playing area and two 'In Goal' areas continuing on behind the 'Try Line' upon which the H-shaped goalposts sit.
The field of play extends into this area, the lower parts of the vertical posts play no purpose other than to hold the upper elements in the air. A 'Try' (roughly equivalent to a Touchdown) can be scored by placing the ball somewhere over the line or by touching the base of the (often padded) posts.
The cross-bar and the verticals upwards of it (towards and bounded at infinity) count as the hard boundary of a scoring area for "conversions" (taken immediately after a try) and other kicks (penalties and drop-goals).
|-
Might be treated as Type 3 (two holes), unless concerned about whether balls kicked through the goals or taking across the try line weave back one or other side of, or between, the lower vertical posts.
Alternately, is a Type 3 for the lower (not more special for scoring than any adjacent lower area) frames, while the open tops (meaningful for scoring purposes) rationalise as topologically irrelevent.
(TL;DR; - It's too long, you may not want to read it...) 162.158.34.213 21:47, 28 May 2022 (UTC)
The extended discussion in the explanation about the issues with "two-holes for football" goes away if the goals are the H-shaped kind rather then the Y-shaped kind. Since the comic specifically states that these fields belong to the Topology Department - and are NOT generalized across all sports fields - then we can use the "two hole" information to deduce that the department's fields have the H-shaped kind...which solves 100% of the confusion and eliminates the long (and excessively intricate) digression about other weird forms of "football" with different topologies. SteveBaker (talk) 13:23, 29 May 2022 (UTC) - agree Boatster (talk) 15:52, 29 May 2022 (UTC)
Is this really explainxkcd? Asking since I don't see the obvious stated anywhere. Hell, the obvious question and last statement of the image isn't even addressed. Why does no one ever want to use the topology department's athletic fields? Its a mystery right? Whats wrong with a soccer field that has a topology like that? It make detecting when the ball crossed the line so much easier. Also, how has no one talked about the geographic/field topology that the last question implies along with the obvious reprecusions (ball roll down hill. stuck in middle. habing to climb. tripping in holes and breaking legs)? Why is everything so freaking high level here? Where the hell is the explanation of the joke's? Something is terribly wrong! 172.71.82.81 17:56, 29 May 2022 (UTC)
- One of the more serious problems with explainxkcd is the well-known phenomenon that explaining a joke often kills the humor. So, quite often, in the course of fully explaining the cartoon - we do indeed shred the actual humor into tiny, tiny fragments. However, we're here to explain it - and that's that.
- I guess the joke is that the topology department are so obsessed with the topological shape of their sport's fields that they have lost the shape and dimensions of the fields - and thereby made them useless for playing actual sports on.
- Two fields that are topologically equivalent are not necessarily capable of being used for playing multiple sports. Swimming on a croquet field - or playing croquet in a swimming pool does not work. 172.69.71.199 18:15, 29 May 2022 (UTC)
- The goal is to explain the joke in laymans terms yaknow, "because your dumb". Since the joke is missed by those outside the fields and don't know how definitions of terms differ in different fields and whatnot. Its the whole purpose. The thing above explains nothing in laymans terms. There is no joke. All there is is an explanation on how field theory and topolgy work and then why the resulting images make sense. Nothing on why this is supposed to be funny. The one thing we actually have to explain at minimum. The joke seems to be that this field which is created for the reasons already described is the actual field we would play on (something completly unaddressed in the explanation above). This could be dangerous with those holes (also unaddressed). And then there is the unadressed question of is this a raised plot of land thats been cut out, or is this all that exist, and kicking the ball off field or falling in a hole goes into a void. This needs to be an explanation for people who are much, much, much dumber. We are not supposed to be explaining field theory, just enough of it to get the joke 162.158.187.124 18:27, 29 May 2022 (UTC)
- explainxkcd was weird, but has definitely been getting weirder, and i also question its reality and worry for if the server breaks without somebody to fix it. i get a lot of reverses and edits that sometimes look like subtle vandalism or political information insertion. i think a lot of people are on twitter, and i think xkcd has an irc chat too. but i'm here for now. 172.70.110.209 09:02, 31 May 2022 (UTC)
Wondering if any topologists understand American football, and if any football fans understand topology. I am a football fan who doesn't understand topology. As requested before, I would like to understand why there is any topological difference in analyzing the American football gameplay and playing field, between H-shaped and Y-shaped goals.
The field-goal-space is functionally a rectangle above the crossbar, and the width between the uprights, but of undefined height, in both the H and Y cases. It is directly above the back line of the endzone for pro and for college football. The one or two supports for the crossbar are irrelevant to gameplay. All supports below the bar would be eliminated, if the engineering problem could be solved. Why does the existence of one vs. two engineering kludges make a critical difference in the number of topological holes?
The endzone, that is, all of the space on the playing field (grass) in front of, and on either side of the goalposts is valid and legal for every player and for the ball on every play, potentially with scoring implications at the termination of the play. Note that the goal posts for pro football were at one time at the back of the endzone, then from 1933 to 1974, on the goal line, and since 1974, at the back of the endzone again. NCAA/college football has had the goalposts at the back of the endzone since 1927.
All of the space above the grass, above the endzone, both under and above the height of the horizontal crossbar, are also legal and valid for play by the players and by the ball on every play. In one case, a play involving a legally kicked field goal, the space above the crossbar and between the uprights, has scoring significance. A field goal has the same name and the same general mechanics in basketball and in American football. In neither case do the engineering contrivances supporting and suspending the goal rectangle (football) or circle (basketball) play a conceptual role in the gameplay. Why, then, do the topologists here in the discussion treat football and basketball differently, and why are H-shaped and Y-shaped goals in football not equivalent? Randall counts both basketball and football as 'two-holers', but the current public Expain xkcd text says that he is wrong for pro and college football. So far as I can tell, pro and college football have both used the Y-goal since 1974 or before. The Y-support for the goalposts is 6.5 feet behind the back of the endzone, and completely outside of the playing field. I look forward to learning something. [unsigned]
- I've edited the first paragraph to make this clearer, but topology is the mathematics which describes a particular aspect of a shape, which ignores many other specifics of shape, size and material. In particular topology pays attention to any place where something can pass through an object, like the holes shown (and places where the object passes through itself are even more interesting). So, while difference between the two supporting poles of the H-support goal and the single pole of the Y-support is irrelevant to gameplay, as far as topology is concerned it is basically the only relevant difference, as two poles supporting a bar form an aperture that things (balls, people) can pass through, and there is one such "hole" in each end.
- I am pretty sure Randall is ignoring all markings on the field and rules of play, considering that the joke is that the topology department is ignoring such important things as size (of volleyball vs high-jump "fields"), positioning (of basketball hoops vs parallel bars) and protrusions (of soccer nets or tetherball stands).172.68.66.37 02:21, 30 May 2022 (UTC)
In describing the shapes for the transcript, amused to find that the ellipses (plural of ellipse, oval) are used to denote ellipses (plural of ellipsis, missing material). Are the etymologies related? 172.68.66.37 02:21, 30 May 2022 (UTC)
The discussion around football type appears to have missed out a fundamental point of terminology, namely that rugby is never called "football". It is one of the variants of football-type games, there is the fact that its codes are governed by the various Rugby Football Unions and Rugby Football Leagues around the world, there's even the fact that it's one of the things referred to by Australians as "footy", which derives from "football"... but isn't actually the word "football". While the word "football" obviously means different things in different contexts, when used on its own, without qualification, it never means "rugby".
Leaving the nets in place on a soccer field which is also used for other activities is not that usual, but it's not unheard of and does make easy sense of the lack of topological holes (and is easily explained by an American's likely unfamiliarity with common soccer-apparatus practice). H-shaped American-football posts have holes bounded by the ground, uprights and crossbar; these holes exist physically and may be described topologically, irrespective of their irrelevance to gameplay. Yorkshire Pudding (talk) 09:24, 31 May 2022 (UTC)
- Call yourself
a pudding, you Yorkshirea Yorkshire, you pudding!?! It is far from uncommon to call the game of 'rugby' by the name of "football" in the real heartlands of the sport... Though I doubt that this terminology has encroached into the US, what with their obsession with body-armour to slow things down and then taking a two-minute breather for every other minute of actual play in their peculiar version of handegg... ;) (I'm not casting aspersions upon their choices of sports, though, I hear they're fairly keen on rounders, which is actually slightly faster paced than even our one-day cricket...) 172.70.162.5 10:20, 31 May 2022 (UTC)- Ah, cobblers. It is uncommon to call anything other than the kicking of a round ball "football", even in the realm of the oval-balled faithful. Ask A. N. Other resident of the West Riding about football - they're not going to think you mean Trinity, Tigers or Hull K.R. Yorkshire Pudding (talk) 23:53, 31 May 2022 (UTC)
Can someone please clarify or remove the parenthesis about "points, in different disconnected topologies"? The fact that loops around a hole cannot be moved to loops that aren't around a hole is one thing, but where do the points or disconnected topologies come in? Or is it a separate concept, of points existing in one topology not existing in another one because there is a hole there? 172.68.146.81 02:51, 1 June 2022 (UTC)
Amused that the citation needed comment on swimming pools being filled with water is not fulfilled by the wikilink for Olympic-sized swimming pools, as that article does not explicitly state anywhere that the pool should be filled with water! 172.68.146.81 03:16, 1 June 2022 (UTC)
To the editor who corrected the "ellipsis" in the transcript, not sure if you're reading the discussion, but it was deliberate and I do know the difference - the whole reason I noticed the ellipses/ellipses double meaning was because I was trying to replace the word "hole", as the rest of the article hadn't yet been updated to clarify the difference between topological holes in a negative space and holes in a solid object. As it stands, "ellipse" is probably the better word for the purposes of describing the picture for the transcript, rather than "ellipsis" for the missing material it is depicting. If I've slipped up anywhere else, please feel free to fix. 172.68.66.63 00:45, 3 June 2022 (UTC)
The fact that the volleyball net creates a hole is absolutely correct. (I'm sure) the configuration is in the rules, including at least the approximate height of the open space under the net. Critically, touching the net is a violation. But temporarily moving under it is not, as long as one doesn't interfere with the opposing team. A net that started from the floor would change the game dramatically, since not even toes could ever legally be extended under it. 172.69.42.91 23:11, 14 November 2022 (UTC)
Except for the long jump community. GetPunnedOn -- GetPunnedOn (talk) 23:23, 21 May 2023 (please sign your comments with ~~~~)

