Difference between revisions of "1591: Bell's Theorem"
(→Explanation: superliminal --> superluminal) |
(added wiki links, corrected one typo) |
||
| Line 10: | Line 10: | ||
{{incomplete|Title Text - Where does "turning off the signal" come in to the explanation of the title text? Still missing lots of wiki links in the entire explanation.}} | {{incomplete|Title Text - Where does "turning off the signal" come in to the explanation of the title text? Still missing lots of wiki links in the entire explanation.}} | ||
| − | {{w|Bell's Theorem|Bell's Theorem}} states "No physical theory of (finitely many) local hidden variables can ever reproduce all of the predictions of quantum mechanics." It says that a theoretical treatment that divides the universe up into separate ("local") systems like this will always discard something about those systems' intercorrelations. | + | {{w|Bell's Theorem|Bell's Theorem}} states "No physical theory of (finitely many) {{w|Local_hidden_variable_theory|local hidden variables}} can ever reproduce all of the predictions of quantum mechanics." It says that a theoretical treatment that divides the universe up into separate ("local") systems like this will always discard something about those systems' intercorrelations. |
"Global hidden variables" are another story: if there is classical information shared across systems (perhaps by superluminal communication) even up to superdeterminism where the universe is just reading off a script, any correlations can be explained away. But this is unsatisfying. | "Global hidden variables" are another story: if there is classical information shared across systems (perhaps by superluminal communication) even up to superdeterminism where the universe is just reading off a script, any correlations can be explained away. But this is unsatisfying. | ||
| Line 19: | Line 19: | ||
===More=== | ===More=== | ||
| − | In quantum mechanics (QM), "measurement" is the process of allowing a small system to interact with its environment in a controlled way. The interaction allows information about the system's state to escape to the environment, producing an "observation." If the measurement apparatus is governed by classical mechanics (impossible in reality, but a very common simplification for the purposes of calculation), then the observation can be thought of as classical information, a bit (yes/no answer) in the simplest case. While the system may have been in any one of infinitely many states before the measurement (each a superposition of classical states), the fact that the measurement must leave it consistent with the classical result means that it can end up in only finitely many states afterwards. This is the "wave-function collapse" of early QM, popularized by Schrödinger's cat, but unrelated to the Heisenberg Uncertainty Principle, with which lay audiences often confuse it. | + | In quantum mechanics (QM), "measurement" is the process of allowing a small system to interact with its environment in a controlled way. The interaction allows information about the system's state to escape to the environment, producing an "observation." If the measurement apparatus is governed by classical mechanics (impossible in reality, but a very common simplification for the purposes of calculation), then the observation can be thought of as classical information, a bit (yes/no answer) in the simplest case. While the system may have been in any one of infinitely many states before the measurement (each a superposition of classical states), the fact that the measurement must leave it consistent with the classical result means that it can end up in only finitely many states afterwards. This is the "wave-function collapse" of early QM, popularized by {{w|Schrödinger's_cat|Schrödinger's cat}}, but unrelated to the Heisenberg {{w|Uncertainty_principle|Uncertainty Principle}}, with which lay audiences often confuse it. |
Modern quantum mechanics acknowledges that the environment is not classical, and that wave-function collapse happens by a (comparatively) gradual process called "decoherence," where information leaving the system is made up for by information coming from the environment that drives the system closer and closer to one of the finitely many states predicted by the simplified model above. (I.e., if a "Schrödinger's cat" is in a half-and-half superposition of the states "dead" and "alive", when its liveness is measured, the ratios of "dead" and "alive" will shift rapidly towards (though not quite reach) 0 and 100% or 100 and 0%. For all but the shortest time scales, the cat's post-measurement state might as well be classical.) | Modern quantum mechanics acknowledges that the environment is not classical, and that wave-function collapse happens by a (comparatively) gradual process called "decoherence," where information leaving the system is made up for by information coming from the environment that drives the system closer and closer to one of the finitely many states predicted by the simplified model above. (I.e., if a "Schrödinger's cat" is in a half-and-half superposition of the states "dead" and "alive", when its liveness is measured, the ratios of "dead" and "alive" will shift rapidly towards (though not quite reach) 0 and 100% or 100 and 0%. For all but the shortest time scales, the cat's post-measurement state might as well be classical.) | ||
| − | Entanglement is a situation where the future outcomes of two or more measurements that would be independent in a classical world are nonetheless correlated. For example, two widely separated electrons could be in a state where, considered individually, each is in a superimposed spin-up/spin-down state, but if one is measured as spin-up, the others will necessarily be measured as spin-down. This is untroubling if the two electrons are modeled as a single system, but strange-seeming if we think of them as separate: how did the measurement of the first electron allow information from the environment around it affect the far-away second electron? It seems like the electrons are communicating, potentially at superluminal speeds, which would violate either relativity or causality. (In actuality, there's a fairly simple proof (see below) that correlations from entanglement can't be used to communicate, and causality and relativity are safe. But that doesn't make the seemingly faster-than-light effects much less of a surprise.) | + | {{w|Quantum_entanglement|Entanglement}} is a situation where the future outcomes of two or more measurements that would be independent in a classical world are nonetheless correlated. For example, two widely separated electrons could be in a state where, considered individually, each is in a superimposed spin-up/spin-down state, but if one is measured as spin-up, the others will necessarily be measured as spin-down. This is untroubling if the two electrons are modeled as a single system, but strange-seeming if we think of them as separate: how did the measurement of the first electron allow information from the environment around it affect the far-away second electron? It seems like the electrons are communicating, potentially at superluminal speeds, which would violate either relativity or causality. (In actuality, there's a fairly simple proof (see below) that correlations from entanglement can't be used to communicate, and causality and relativity are safe. But that doesn't make the seemingly faster-than-light effects much less of a surprise.) |
| − | One can try to address these concerns by considering 'local hidden variables', classical properties of a local system (like a single electron) that could have been observed but were not. For example, perhaps a classical part of the electrons' state lets them "agree" on a future classical state at the moment | + | One can try to address these concerns by considering 'local hidden variables', classical properties of a local system (like a single electron) that could have been observed but were not. For example, perhaps a classical part of the electrons' state lets them "agree" on a future classical state at the moment they are entangled, and then they just reveal that state in the future. But this becomes unwieldy: there are infinitely many possible future observations the electrons would have to agree on, and it seems difficult to do this without infinitely many local hidden variables. |
===Title Text=== | ===Title Text=== | ||
| − | The real No-Communication Theorem states that although determination of the state of one half of an entangled pair immediately determines that of the other half, however far away it may be, there's no way for the observer of the other half to see if he's the first to find out the state or whether it'd already been determined by the first observer. Thus, no information travels from one observer to the other. Randall's version is recursive. It hypothesises a method of communication whereby somebody misunderstanding the no-communication theorem (which also happens faster than the speed of light) could function as the reception of a faster-than-light signal. However, it goes on to point out that turning the signal off requires clearing up the confusion which takes much, much longer, thus neatly restoring the normality of slower than light communication. | + | The real {{w|No-communication_theorem|No-Communication Theorem}} states that although determination of the state of one half of an entangled pair immediately determines that of the other half, however far away it may be, there's no way for the observer of the other half to see if he's the first to find out the state or whether it'd already been determined by the first observer. Thus, no information travels from one observer to the other. Randall's version is recursive. It hypothesises a method of communication whereby somebody misunderstanding the no-communication theorem (which also happens faster than the speed of light) could function as the reception of a faster-than-light signal. However, it goes on to point out that turning the signal off requires clearing up the confusion which takes much, much longer, thus neatly restoring the normality of slower than light communication. |
==Transcript== | ==Transcript== | ||
Revision as of 09:03, 19 October 2015
Explanation
| |
This explanation may be incomplete or incorrect: Title Text - Where does "turning off the signal" come in to the explanation of the title text? Still missing lots of wiki links in the entire explanation. If you can address this issue, please edit the page! Thanks. |
"Global hidden variables" are another story: if there is classical information shared across systems (perhaps by superluminal communication) even up to superdeterminism where the universe is just reading off a script, any correlations can be explained away. But this is unsatisfying.
The prefered resolution of the paradox is not to insist (as early physicists did) that the universe's state is a collection of bits (classical information), but treat it as a collection of qubits (quantum information).
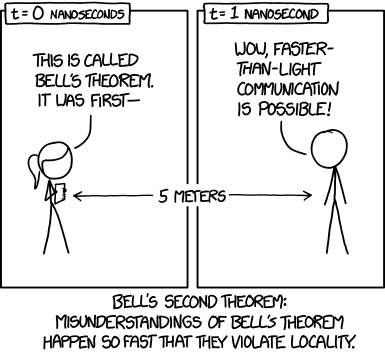
Ponytail begins reading Bell's theorem to Cueball, who is standing 5 meters away. Cueball responds with a misunderstanding of Bell's Theorem in 1 nanosecond. The speed of light in a vacuum is 299,792,458 meters per second. In one nanosecond, the light from Ponytail would only have traveled 0.3 meters, thus Cueball misunderstands Bell's Theorem faster than the light from Ponytail reading the theorem can reach him, which implies that faster-than-light communication occurred to set up the misunderstanding.
More
In quantum mechanics (QM), "measurement" is the process of allowing a small system to interact with its environment in a controlled way. The interaction allows information about the system's state to escape to the environment, producing an "observation." If the measurement apparatus is governed by classical mechanics (impossible in reality, but a very common simplification for the purposes of calculation), then the observation can be thought of as classical information, a bit (yes/no answer) in the simplest case. While the system may have been in any one of infinitely many states before the measurement (each a superposition of classical states), the fact that the measurement must leave it consistent with the classical result means that it can end up in only finitely many states afterwards. This is the "wave-function collapse" of early QM, popularized by Schrödinger's cat, but unrelated to the Heisenberg Uncertainty Principle, with which lay audiences often confuse it.
Modern quantum mechanics acknowledges that the environment is not classical, and that wave-function collapse happens by a (comparatively) gradual process called "decoherence," where information leaving the system is made up for by information coming from the environment that drives the system closer and closer to one of the finitely many states predicted by the simplified model above. (I.e., if a "Schrödinger's cat" is in a half-and-half superposition of the states "dead" and "alive", when its liveness is measured, the ratios of "dead" and "alive" will shift rapidly towards (though not quite reach) 0 and 100% or 100 and 0%. For all but the shortest time scales, the cat's post-measurement state might as well be classical.)
Entanglement is a situation where the future outcomes of two or more measurements that would be independent in a classical world are nonetheless correlated. For example, two widely separated electrons could be in a state where, considered individually, each is in a superimposed spin-up/spin-down state, but if one is measured as spin-up, the others will necessarily be measured as spin-down. This is untroubling if the two electrons are modeled as a single system, but strange-seeming if we think of them as separate: how did the measurement of the first electron allow information from the environment around it affect the far-away second electron? It seems like the electrons are communicating, potentially at superluminal speeds, which would violate either relativity or causality. (In actuality, there's a fairly simple proof (see below) that correlations from entanglement can't be used to communicate, and causality and relativity are safe. But that doesn't make the seemingly faster-than-light effects much less of a surprise.)
One can try to address these concerns by considering 'local hidden variables', classical properties of a local system (like a single electron) that could have been observed but were not. For example, perhaps a classical part of the electrons' state lets them "agree" on a future classical state at the moment they are entangled, and then they just reveal that state in the future. But this becomes unwieldy: there are infinitely many possible future observations the electrons would have to agree on, and it seems difficult to do this without infinitely many local hidden variables.
Title Text
The real No-Communication Theorem states that although determination of the state of one half of an entangled pair immediately determines that of the other half, however far away it may be, there's no way for the observer of the other half to see if he's the first to find out the state or whether it'd already been determined by the first observer. Thus, no information travels from one observer to the other. Randall's version is recursive. It hypothesises a method of communication whereby somebody misunderstanding the no-communication theorem (which also happens faster than the speed of light) could function as the reception of a faster-than-light signal. However, it goes on to point out that turning the signal off requires clearing up the confusion which takes much, much longer, thus neatly restoring the normality of slower than light communication.
Transcript
[First frame captioned: t = 0 nanoseconds]
Ponytail, holding a piece of paper and facing to the right: This is called Bell's Theorem. It was first–
[A double-headed arrow links the characters in the two frames. The arrow is labelled "5 meters".]
[Second frame captioned: t = 1 nanosecond]
Cueball, facing to the left towards Ponytail: Wow, faster-than-light communication is possible!
Caption: Bell's Second Theorem: Misunderstandings of Bell's Theorem happen so fast that they violate locality.
Discussion
I'm sure some people here have this memorised, but light travels just under 30 centimetres in a nanosecond. For our Metric-ally challenged friends, that's about one foot – so 5 metres takes around 16.67 nanoseconds. I leave the comic explanation to smarter people than me. Paddles (talk) 13:02, 16 October 2015 (UTC)
- I have seen Admiral Grace Hopper demonstrate this with approximately foot-long lengths of wire representing "light-nanoseconds". It's accurate to one part in 50 (although not as accurate as the one-part-in-1000 "30 centimeters" measurement). PsyMar (talk) 20:33, 16 October 2015 (UTC)
- The problem with that nifty rule-of-thumb is that it is technically correct, but practically useless. The 30cm/ns is for light in a vacuum. For an electrical signal in a wire (or light in a fibre, for that matter) the effective speed is roughly 20cm/ns. -- Popup (talk) (please sign your comments with ~~~~)
The comic only shows that the two characters are 5m apart at chest level. What if there was a miniature wormhole or distortion in time in a separate area, making this seemingly "FTL" communication scientifically possible? Forrest (talk)14:19, 16 October 2015 (UTC)
For an explanation of Bell's theorem in the words of the man himself, and targeted at an educated lay audience, this is essential reading: https://cds.cern.ch/record/142461/files/198009299.pdf 162.158.35.36 16:22, 16 October 2015 (UTC) : Tim B posting as Anon
Wow, the explanation needs some explaining. Can the first part about quantum mechanics be simplified, moved, or have something clearer put in front of it? I don't feel up to the task, but the section is not very helpful. -DanB (talk) 17:32, 16 October 2015 (UTC)
- Yeah, the explanation isn't actually an explanation at all. Can someone who understands Bell's Theorem write an explanation for the joke in the comic? The current explanation appears to be a non sequitorial digression. I'm really curious as to what the actual joke is about. 108.162.249.155 04:20, 9 March 2016 (UTC)
In the widely separated electrons section, isn't it necessary that the two electrons measured be from the same source? If so, the explanation could use that small edit, but I'm not sure I'm remembering right. Miamiclay (talk) 05:35, 17 October 2015 (UTC)
- Yes.
I think this whole explanation is suffering from "Bell's second theorem".
Can anyone cite an experiment or proof that *altering* the state of one half of an entangled electron pair *after* they have been separated to a significant distance has any effect upon the other half? So far as I have learned, the two electrons in question are driven to opposite states by close proximity: When separated, they maintain cyclical synchrony until the state of one electron is measured. Environmentally induced state changes have not been shown to propagate between entangled particles after they are separated; They simply retain oppositional synchrony until disentangled by observation (or other interference). Any information derived was imparted at the point of entanglement, or during transit, or by measurement. Introducing new information (state change) to one half of an entangled pair after separation interrupts the synchronous effect, disrupting the entanglement. This is not useful from a communications standpoint.
- Correct, there is nothing that changes about the second particle when the first particle is measured
Nothing in quantum mechanics actually violates classical mechanics; Rather, quantum mechanics acknowledges that our ability to measure a near-infinite (but still finite) set of variables is limited by the effect of our own observation & by our inability to quantify all relevant variables prior to comparison. Thus "quantum uncertainty" & wave function collapse are merely an admission that any data set is necessarily incomplete, while reserving the possibility of predicting deterministic outcomes by reasoned observation of the limited data available.
At least, that's what the cat told me. 108.162.221.47 06:54, 17 October 2015 (UTC)
- That is exactly what Bell's theorem states and what the experiments behind it showed. It is a bit technical, but the best layman description I have seen was on Ars Technica: http://arstechnica.com/science/2010/01/a-tale-of-two-qubits-how-quantum-computers-work/
162.158.92.91 09:41, 17 October 2015 (UTC)
The first rule of the No Communication Theory is that you don't talk about the No Communication Theory. -Pennpenn 108.162.250.162 22:44, 18 October 2015 (UTC)
"This means that any complete description..." - isn't this exactly the misunderstanding the comic is making fun of? I don't think one can make such an inference without first unscientifically presupposing some interpretations of QM to be correct.
I don't see how quantum mechanics would make "free will" possible and how superdeterminism is uncomfortable. It's still physics, just harder to predict. If you want a true free will—not controlled by the physical calculations of our brains—you need an entity detached from the physical world; a soul. That's a subject for religion and not something for scientists to be concerned with. At least not at this time. If souls do exist then science might detect them one day, but for now they are just fantasy and I doubt they will be found in quantum mechanics. If souls control anything, then the tiniest interaction is basically a miracle and not something science can predict. If souls exist in quantum mechanics, then every particle has a soul and it would be ridiculous to think that each person's "free will" was controlled by a single entity rather than the sum of several billion entities. That's just my two cents. I'm no scientist. I'm just baffled by people being uncomfortable with their free will not being an undeterministic force affecting the universe from the outside, as if it would make their experience of life any different whether it is or not. Kapten-N (talk) 07:14, 1 October 2024 (UTC)