Difference between revisions of "2936: Exponential Growth"
(Full quantity rather than just the last square) |
m (→Explanation) |
||
| Line 14: | Line 14: | ||
In this strip Black Hat begins by demonstrating the {{w|exponential growth}}, using a variation of the {{w|wheat and chessboard problem}}, a classic demonstration of this mathematical principle. Exponential growth involves an initial quantity being multiplied by any number greater than one again and again. It can cause small numbers to compound into very large numbers faster than might be intuitive. This principle is important in a number of real life applications, ranging from biological growth to inflation to reaction kinetics. | In this strip Black Hat begins by demonstrating the {{w|exponential growth}}, using a variation of the {{w|wheat and chessboard problem}}, a classic demonstration of this mathematical principle. Exponential growth involves an initial quantity being multiplied by any number greater than one again and again. It can cause small numbers to compound into very large numbers faster than might be intuitive. This principle is important in a number of real life applications, ranging from biological growth to inflation to reaction kinetics. | ||
| − | The earliest versions of this story come from India and involve a man (the inventor of chess, in some tellings), being offered a reward by a king, and asking that a single grain of wheat be placed on the first square of a chessboard, two on the second, and each subsequent square having twice as many grains as the one before. In the story, the king generally laughs off such a reward as being trivial, but soon learns that the reward would be impossible to pay. Since a chessboard contains 64 squares, the final square would contain 2^63 (approximately 9.2 quintillion) grains. This would be around 600 billion tonnes of wheat (even in modern times, this is more than 750 years of global wheat output). Worse, that's just for the final square -- adding up all the squares would require about double that (approximately 18.4 quintillion | + | The earliest versions of this story come from India and involve a man (the inventor of chess, in some tellings), being offered a reward by a king, and asking that a single grain of wheat be placed on the first square of a chessboard, two on the second, and each subsequent square having twice as many grains as the one before. In the story, the king generally laughs off such a reward as being trivial, but soon learns that the reward would be impossible to pay. Since a chessboard contains 64 squares, the final square would contain 2^63 (approximately 9.2 quintillion) grains. This would be around 600 billion tonnes of wheat (even in modern times, this is more than 750 years of global wheat output). Worse, that's just for the final square -- adding up all the squares would require about double that (2^64-1 which is approximately 18.4 quintillion grains). In some versions of the story the man is executed for embarrassing the king, in others, he's rewarded for his cleverness. |
[[Black Hat]] initially appears to be using this example (with rice, rather than wheat), to demonstrate a mathematical principle, but actually turns out to be using it to "win" a chess match by covering the chess board in rice until his opponent quits out of frustration. Naturally, despite his claims that it's "nearly impossible to counter", under the International Chess Federation (FIDE)'s [https://www.fide.com/FIDE/handbook/LawsOfChess.pdf Laws of Chess], this would be illegal on several levels, as deliberately distracting or annoying your opponent is violation, as is deliberately displacing the chess pieces. Black Hat being Black Hat, he likely simply doesn't care, and counts it as a win when his opponent stomps off out of annoyance. | [[Black Hat]] initially appears to be using this example (with rice, rather than wheat), to demonstrate a mathematical principle, but actually turns out to be using it to "win" a chess match by covering the chess board in rice until his opponent quits out of frustration. Naturally, despite his claims that it's "nearly impossible to counter", under the International Chess Federation (FIDE)'s [https://www.fide.com/FIDE/handbook/LawsOfChess.pdf Laws of Chess], this would be illegal on several levels, as deliberately distracting or annoying your opponent is violation, as is deliberately displacing the chess pieces. Black Hat being Black Hat, he likely simply doesn't care, and counts it as a win when his opponent stomps off out of annoyance. | ||
Revision as of 14:20, 23 May 2024
| Exponential Growth |
 Title text: Karpov's construction of a series of increasingly large rice cookers led to a protracted deadlock, but exponential growth won in the end. |
Explanation
| |
This explanation may be incomplete or incorrect: Created by a 2^64TH ITERATION OF A BOT - Please change this comment when editing this page. No mention of the title text yet. If you can address this issue, please edit the page! Thanks. |
In this strip Black Hat begins by demonstrating the exponential growth, using a variation of the wheat and chessboard problem, a classic demonstration of this mathematical principle. Exponential growth involves an initial quantity being multiplied by any number greater than one again and again. It can cause small numbers to compound into very large numbers faster than might be intuitive. This principle is important in a number of real life applications, ranging from biological growth to inflation to reaction kinetics.
The earliest versions of this story come from India and involve a man (the inventor of chess, in some tellings), being offered a reward by a king, and asking that a single grain of wheat be placed on the first square of a chessboard, two on the second, and each subsequent square having twice as many grains as the one before. In the story, the king generally laughs off such a reward as being trivial, but soon learns that the reward would be impossible to pay. Since a chessboard contains 64 squares, the final square would contain 2^63 (approximately 9.2 quintillion) grains. This would be around 600 billion tonnes of wheat (even in modern times, this is more than 750 years of global wheat output). Worse, that's just for the final square -- adding up all the squares would require about double that (2^64-1 which is approximately 18.4 quintillion grains). In some versions of the story the man is executed for embarrassing the king, in others, he's rewarded for his cleverness.
Black Hat initially appears to be using this example (with rice, rather than wheat), to demonstrate a mathematical principle, but actually turns out to be using it to "win" a chess match by covering the chess board in rice until his opponent quits out of frustration. Naturally, despite his claims that it's "nearly impossible to counter", under the International Chess Federation (FIDE)'s Laws of Chess, this would be illegal on several levels, as deliberately distracting or annoying your opponent is violation, as is deliberately displacing the chess pieces. Black Hat being Black Hat, he likely simply doesn't care, and counts it as a win when his opponent stomps off out of annoyance.
Garry Kasparov and Antoly Karpov are both Russian chess grandmasters and former world champions. The two men famously competed for the world championship in the 1980s. The Kasparov gambit is an opening move in chess. The title text implies that Kasparov actually tried this method on Karpov, who attempted to consume all the rice with "increasingly large rice cookers", but eventually couldn't keep up. While this is obviously fictional, it fits with the principle of exponential growth. If exponential growth is unrestricted, it will eventually grow beyond the constraints of anything that could plausibly be built to contain it.
Needless to say, this process would be impossible to do. [citation needed]
Transcript
| |
This transcript is incomplete. Please help editing it! Thanks. |
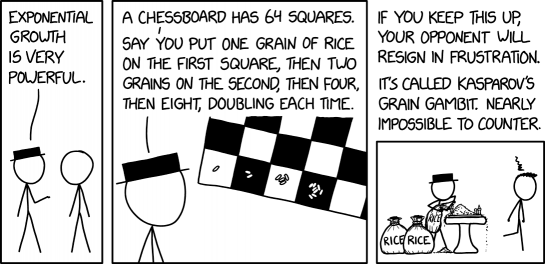
- [Black Hat is talking to Cueball standing next to him.]
- Black Hat: Exponential growth is very powerful.
- [Closeup on Black Hat. Next to him is an image of the lower left part of a chessboard. The four leftmost squares in the bottom row have grains of rice on them -- one, two, four, and eight grains respectively.]
- Black Hat: A chessboard has 64 squares.
- Black Hat: Say you put one grain of rice on the first square, then two grains on the second, then four, then eight, doubling each time.
- [Black Hat has emptied a bag of rice on a chessboard. There are several bags next to him and a pile of rice already on the table. A frustrated Hairy is walking away, fists clenched.]
- [Caption above panel, representing Black Hat continuing to speak:]
- If you keep this up, your opponent will resign in frustration.
- It's called Kasparov's Grain Gambit. Nearly impossible to counter.
Discussion
If that's done by each of your moves being to add one (more) grain to the board, the game would last quite a while. Even with reduced time-limits on the game-clock. 172.70.91.154 21:27, 22 May 2024 (UTC)
Hmmm. Interesting. 172.69.58.203 21:31, 22 May 2024 (UTC)
First transcript! Hope it's good.Danger Kitty (talk) 21:36, 22 May 2024 (you only ~~~ed, it looks like...)
Total of 2^64 - 1 ≈ 1.8 x 10^19 grains of rice. If a grain of rice averages 30 mg, then that's 5.5 x 10^14 kg of rice. That's around the mass of Lake Erie. 172.71.223.56 21:38, 22 May 2024 (UTC)
The legend about the chess board and doubling the grain placed on each square is researched here: https://history.stackexchange.com/questions/5992/what-is-the-origin-of-the-wheat-and-chessboard-legend 172.71.150.113 21:50, 22 May 2024 (UTC)~
The rice is on the side or the board is turned wrong. 172.70.115.17 (talk) 23:13, 22 May 2024 (please sign your comments with ~~~~)
- ...not sure what you mean here. (Also, do sign your contributions.) 172.70.162.186
- The white square always goes on your right corner so this border is sideways (assuming we're looking at it head on, which seems likely) Apollo11 (talk) 23:35, 22 May 2024 (UTC)
- a1 is a dark square, so wherever the one grain of rice is, it can't be a1. 172.71.102.35 08:41, 23 May 2024 (UTC)
With all those zeros in the values given for row eight i assume we are looking at the limitations of someones calculation skills/calculator... last I checked 5 was not a factor of any 2^n value? 172.70.80.246 00:13, 23 May 2024 (UTC)
- I think we don't need this part at all. If we really want to illustrate the numbers we could simply use the illustration from here: https://en.wikipedia.org/wiki/Wheat_and_chessboard_problem#Second_half_of_the_chessboard Elektrizikekswerk (talk) 07:15, 23 May 2024 (UTC)
That doesn't look like Hairy in the final panel. Is it a Kasparov caricature? Nitpicking (talk) 02:12, 23 May 2024 (UTC)
- I agree it is not the standard Hairy. Since this is Kasparovs gambit and Karpov tried to counter it, then it should be Karpov that walks out! Even though it is not Kasparaov but Black Hat that used the gambit. --Kynde (talk) 08:59, 24 May 2024 (UTC)
- Maybe it's the same Cueball from the first panel, but he's had to wait so long while Black Hat fetched all the rice that his hair grew out.172.70.160.249 13:32, 24 May 2024 (UTC)
I have to wonder if this comic is related to the Casablanca Chess Tournament that took place this past week, where 4 top-ranked players competed by playing a series of real historical games starting from the middle of each game. Magnus Carlsen won the tournament, which also included Hikaru Nakamura, Viswanathan Anand, and Bassem Amin. Ianrbibtitlht (talk) 04:38, 23 May 2024 (UTC)
Russia pulling out of Black Sea agreement has been labelled "grain gambit" --172.71.131.158 06:36, 23 May 2024 (UTC)
Trivia: 1. e2–e4 c7–c5 2. Sg1–f3 e7–e6 3. d2–d4 c5xd4 4. Sf3xd4 Sb8–c6 5. Sd4–b5 d7–d6 6. c2–c4 Sg8–f6 7. Sb1–c3 a7–a6 8. Sb5–a3 d6-d5!? is the Kasparov Gambit, see Wiki. 172.71.160.30 08:56, 23 May 2024 (UTC)
This is a completely normal amount of rice. I eat this much grain daily. Psychoticpotato (talk) 13:21, 23 May 2024 (UTC)
Counter with Tree countergambit. plant tree(1) seeds in the first square and tree(2) on the next square then tree(3) in the next square. Nobody has found out what happens afterwards. 172.70.131.212 (talk) 14:25, 23 May 2024 (please sign your comments with ~~~~)
So, out of curiosity, how many grains of rice can you actually fit on an average chess board square? Or maybe, how big would a chessboard have to be in order for the rice to fit on top of every square without overflowing? 172.69.91.144 22:13, 23 May 2024 (UTC)
- Assuming that its a standard size and it can stack up around 10 cubic inches upwards about 4117267200 grains Apollo11 (talk) 03:08, 24 May 2024 (UTC)
- Judging by this, I reckon if you were really, really patient you might just about corral the 2048 on square 12 to stay within the bounds without additional housing, but you'd have no hope with the 13th.172.70.90.98 14:25, 24 May 2024 (UTC)
- This is super cool. And helpful. I got the density of rice from this and tried to calculate the size of a chess board that could contain the nine quintillion grains of rice on the last square. Assuming the rice forms a cone with a 30° slope, one would need a chess board roughly the size of Colombia (1073296km² for the whole board). Can anyone confirm?172.69.91.165 10:56, 25 May 2024 (UTC)
- I covered Columbia in rice, and can confirm your hypothesis. Though a small amount spilled onto the streets of Tulcan, Ecuador. These Are Not The Comments You Are Looking For (talk) 02:48, 26 May 2024 (UTC)
- That's a neat trick. Especially as there are few Columbias that are adjacent to Ecuador... Probably why it hasn't made the news, with the geopolitical confusion as to what happened where. ;) 172.70.85.31 08:59, 26 May 2024 (UTC)
- If you are willing to trust Google Maps, you can confirm that Tulcan, Ecuador is less than 10km from the border of Columbia. These Are Not The Comments You Are Looking For (talk) 02:39, 27 May 2024 (UTC)
- That's Colombia, not Columbia, I think is the point. 172.70.162.18 10:11, 27 May 2024 (UTC)
- If you are willing to trust Google Maps, you can confirm that Tulcan, Ecuador is less than 10km from the border of Columbia. These Are Not The Comments You Are Looking For (talk) 02:39, 27 May 2024 (UTC)
- That's a neat trick. Especially as there are few Columbias that are adjacent to Ecuador... Probably why it hasn't made the news, with the geopolitical confusion as to what happened where. ;) 172.70.85.31 08:59, 26 May 2024 (UTC)
- I covered Columbia in rice, and can confirm your hypothesis. Though a small amount spilled onto the streets of Tulcan, Ecuador. These Are Not The Comments You Are Looking For (talk) 02:48, 26 May 2024 (UTC)
- This is super cool. And helpful. I got the density of rice from this and tried to calculate the size of a chess board that could contain the nine quintillion grains of rice on the last square. Assuming the rice forms a cone with a 30° slope, one would need a chess board roughly the size of Colombia (1073296km² for the whole board). Can anyone confirm?172.69.91.165 10:56, 25 May 2024 (UTC)
Also, I noticed everyone here seems to have an ip in the 172.69.0.0 to 172.71.255.255 range, but I just checked and that's not even my ip address at the moment. What's that about? Does the wiki mask our actual ip addresses? 172.69.90.110 22:29, 23 May 2024 (UTC)
- Not the wiki, but the gateways to the wiki that help with load-balancing and related connection issues. And you'll also see some IPs in the 141.x.y.z range, and others. I usually am in 171.[69-71].y.z range, but between one contribution another I might be anywhere.
- It's a known thing, for better or worse. Ultimately, there are behind-the-scenes details that would know the 'true' origin of everyone (give or take what load-balancing your own ISP also does at your side of the connection), but it's left obscured from our more plebian eyes.
- Getting a username will also remove the wider and more general geographic potshots someone can make a out your origin (the gateways seen to be used are likely to reveal at least your continent, if anyone's bothered), but I never saw the need.
- ...now. I wonder under what range will the following put me..? => 172.69.194.96 23:34, 23 May 2024 (UTC) 8) Postscript: I first quickly used Preview, and I actually got the 141.range, then posted for real and got the 172s. About ten seconds between the two 'postings'. Hah! 141.101.98.129 23:36, 23 May 2024 (UTC)
"If exponential growth is unrestricted, it will eventually grow beyond the constraints of anything that could plausibly be built to contain it." - Given that the increase in rice grains is, itself, not plausible, I see no reason why the growth in size of rice cookers needs to be plausible either.141.101.98.119 09:59, 24 May 2024 (UTC)
- I was tempted to add something about square/cube-law (not quite applicable, as there'll be a smidgen of cubing as you raise the square-area of container material, etc, but along tbose lines), but that of course makes the implausibility threshold of the cookers higher than the same threshold of rice (everything else being equal). So then you're on to the heat-penetration abilities (after a while, the outer rice is overcooked, when the innermost rice has barely felt the heat). And that leads me to believe that something like a rotary kiln design might be best adopted (external heat, internalised water delivery, properly tuned, and could even be effectively pressurised with the right cycling addons to either end) to just accept rice in at a constant rate and produce perfectly cooked rice at the commensurate output rate. Of course, exponential increase in feed would then require exponential increase in parallel rotary-cookers to handle it, but starting at an already more efficient/controllable mass-cooking process than merely upscaling a traditional pot-style cooker. 172.71.242.220 11:09, 24 May 2024 (UTC)
This is easily defeated. Simply counter by placing one goose on the 64th square, two geese on the 63rd, and so on. They'll quickly deal with the rice situation.172.70.163.120 13:39, 24 May 2024 (UTC)
- But then you need to add an increasing number of foxes starting at the first square to deal with the geese.Mathmannix (talk) 19:27, 24 May 2024 (UTC)
- And good luck taking the whole setup across a river with just a small boat! 172.69.79.165 22:43, 24 May 2024 (UTC)
Correct me if I'm wrong, but assuming that the chess board is 20 inches square, the rice being stacked into a pyrimid 15 inches high, then it only works out to 1.7x10^16 kg/m^3, which, according to Wolfram, is dwarfed by the density of a Neutron Star, much less a black hole. So is there some other reason the explanation claims it will become a black hole? Or was it just wrong Xkcdjerry (talk) 04:17, 25 May 2024 (UTC)
'If someone asking for too much wheat, hit him on the head with chessboard.'--Liu Qi, an emperor in Han dynasty, China 799571388 (talk) 05:23, 2 July 2024 (UTC)
