1964: Spatial Orientation
| Spatial Orientation |
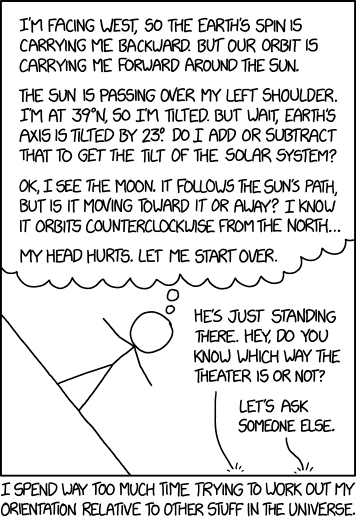 Title text: Here, if you know the number of days until the vernal equinox, I can point you to the theater using my pocket Stonehenge. |
Explanation[edit]
Location in space is always relative, as we cannot observe empty space itself and find an absolute location. Planets are subject to different types of motion, including rotation, precession, and others.
- The Earth (rotation)
Cueball starts by stating that as he is facing west, the Earth's spin will be carrying him backwards. Except at the poles, everything on Earth's surface is being rotated to the east, "toward" the rising Sun in the east or "away" from the setting Sun in the west.
On the equator, Earth's spin is about 464 meters per second (with 464 m being 1/60 of 1/60 of 1/24 of Earth's equatorial circumference of 40070 km, based on the number of seconds in a day, ignoring the difference between sidereal and ephemeris days). So, on the equator at sunrise, on the day of a March or September equinox, this spin, by itself, would take someone toward the Sun at about 464 meters per second.
This spin would be slower than 464 m/s at 39 degrees North. The average radius of the Earth is 6371 km. This means that the distance from a line between the poles through the center of the Earth to a point on Earth's surface at 39°N is approximately 6371 km times the cosine of 39° (0.68 radians), which is 4951 km. So, the distance around the Earth along the 39° latitude "line" is 2π times 4951 km, which is about 31,109 km. (This estimate ignores the oblateness of the Earth.) The rotation of the Earth on its axis would transport points on Earth at 39° latitude to the east at 360 meters per second (1/60 of 1/60 of 1/24 of 31,109). Determining how the direction that is currently east for Cueball is oriented relative to the Sun and the solar system depends on some of the issues Cueball identifies later.
- The Earth (orbit)
Cueball then seemingly corrects himself in his head, having accounted for the fact that the Earth is also revolving around the Sun.
The Earth's orbit around the Sun is counter-clockwise, when viewed from above the North Pole looking down. Earth's counterclockwise orbit around the Sun means that, for most latitudes, the direction the Earth is moving around the Sun corresponds roughly to west at noon, and east in the middle of the night. The Earth is spinning, so "east" from any given location on the surface is not always the same direction relative to the Sun.
The speed of the Earth's orbit around the Sun depends on the time of year. The Earth moves faster around the Sun when it is closest to the Sun in early January, and slower when it is far away in early July (which may be counterintuitive to those in the northern hemisphere). However, Earth's average orbital speed is reportedly about 29.78 kilometers per second, with Earth's average distance from the Sun being a bit less than 150 million kilometers. Earth's orbit around the Sun is nearly circular, with an eccentricity of just 0.0167.
- Earth's tilted axis
Cueball knows that the Earth's axis is tilted (by 23°) relative to its orbit around the Sun and knows that he is 39° north of the equator, but is unsure how to combine this information to figure out his orientation relative to the plane of the solar system.
The Earth’s orbit around the Sun, under Keplerian assumptions, is an ellipse, which lies within a plane. Furthermore, the entire solar system, to some extent, lies within a plane, since the orbital inclinations of Mars and the gas and ice giants are within 2½° of Earth’s and the orbital inclinations of a major body in the solar system (such as a planet) rarely, if ever, varies from that of another by more than 8°. With the exception of Eris, all planets and dwarf planets have an orbital inclination within about 30° of Earth’s.
Cueball is attempting to determine where the plane of the solar system lies with regard to him. Ignoring any possible difference between Earth’s orbit and this plane, and assuming that Cueball is standing on flat ground, the angle between the line from the center of the Earth through Cueball (which runs through his body parallel to his legs and spine if he is standing straight up) and the plane of the solar system can be expressed in terms of two angles: the angle between the plane of Earth’s equator and the solar plane, and the angle between the Earth’s equatorial plane and the vertical line through Cueball. Cueball is at 39°N, so if Cueball is standing straight up, the angle between the plane of the Earth’s equator and the long axis of his body is also 39°. As stated in the comic, Earth’s axis is currently tilted by about 23.4° (an amount which is very slowly decreasing as part of a 41,000 year cycle).
Cueball is trying to determine whether to add together 39° and 23° to get the angle between himself and the solar system’s plane or subtract them. The answer depends on the time of day and the time of year. On the day of the summer solstice in the northern hemisphere (around June 21), the north pole is tilted toward the Sun, so at the longitude that is currently experiencing solar noon, the solar plane passes through a point that is 23° north of the equator. So, if it is solar noon on the summer solstice, Cueball should subtract the angles to find that the direction his body is pointing is roughly 16° away from the solar plane. If he were to somehow lean so that he could tilt his body 16° to the south, the solar plane would pass through the vertical axis of his body and his scalp would be pointed directly toward the Sun. On the other hand, on the day when the northern hemisphere is experiencing the winter solstice (around December 21), the northern hemisphere is pointing away from the Sun, so at solar noon on that day, he would add the angles together to find that his vertical stance is 62° away from the plane of the solar system. (The Sun is never truly directly overhead at latitudes further from the equator than 23.4°. At arctic latitudes that are less than 23.4° from the north pole – more than 67° north of the equator - the Sun is not visible on the day of the winter solstice even when it is noon.)
If it is not a solstice day, or if it is not noon, the calculations could become more complicated. The comic was uploaded roughly two weeks before the northern hemisphere’s spring equinox. Cueball notices that the Sun is “passing over his left shoulder” as he faces west. At temperate latitudes in the northern hemisphere, the Sun would be to the left of a person facing west around midday almost any time of year, although how many degrees to the left depends on the calculations discussed above.
An easier way to identify a line that is aligned with the solar plane would be to simply point directly at the Sun (without hurting his eyes). Since the distance between Cueball and the center of the Earth is minuscule compared to the distance between the Earth and the Sun, if he simply points directly at the Sun (preferably without looking directly at it), his arm and finger will be pointing in a direction that is basically parallel to the line connecting the Earth and Sun, which obviously lies on the plane of the Earth's orbit. The Earth's position will have changed minimally in the eight minutes it took the Sun's light to reach Earth, so the apparent direction to the Sun matches the actual direction. However, this will only provide one line that lies on the plane of the solar system and a line is insufficient to uniquely identify a plane.
- The Moon
Cueball knows about the Moon's path across the sky and knows that its orbit around the Earth appears counter-clockwise when viewed from above the North Pole, but is confused about whether the Moon is moving toward the Sun or away from it.
Like the Earth, the Moon, when viewed from above Earth’s North Pole, both orbits counterclockwise and rotates on its axis counterclockwise (with equal rotational periods such that the same side of the Moon always faces us). (In fact, almost every body in the Solar System both orbits the body it is orbiting counterclockwise and spins on its axis counterclockwise, with the rotational axes of Venus and Uranus being major exceptions.)
A new moon happens when the Moon is closer to the Sun than the Earth is, thus casting the near side of the Moon in darkness because it is the far side of the Moon that is facing the Sun. Conversely, a full moon happens when the Moon is on the other side of the Earth from the Sun; this is why a lunar eclipse can only occur during a full moon. In that sense, it could be said that the Moon is moving perpendicular to the line between it and the Sun at the time of the full moon and the new moon, moving toward the Sun after the full moon until the next new moon, and moving away from the Sun after the new moon until the next full moon.
In another sense, since the Moon is orbiting the Earth and the Earth’s orbit around the sun is elliptical, it could be said that the Moon is getting closer to the Sun whenever Earth is moving toward its perihelion, the point in its orbit that is closest to the Sun, around January 2 to January 5, and moving away as the Earth moves toward its aphelion, the point in its orbit that is furthest from the Sun, around July 3 to July 6. (Yes, the Earth is closest to the Sun in January, despite what those in the northern hemisphere who are tilted away from the Sun at that time may think.) In yet another sense, since the Moon follows the path of the Earth, and the Earth’s orbit around the Sun is roughly circular, and the instantaneous motion of an object in a circular orbit is always perpendicular to the radius connecting it to the orbited body, it could be said that the Moon is always moving perpendicular to the line connecting the Earth and Sun, which is at most a fraction of a degree away from the line connecting the Moon and the Sun.
The semi-major axis of the Moon’s orbit around the Earth (the furthest distance between the Moon and the center of its orbit) is 384,400 km. Compared to the semi-major axis of Earth’s orbit around the Sun, which is 149,600,000 km, the axis of the Moon’s orbit is only 0.26% as large. The Moon’s orbital period is 27.3 days, but its synodic period (the time between full moons; the time it takes the Moon to reappear at the same point in the sky) is 29.5 days.
Cueball internally attempts to orient himself amidst the galactic chaos but is confused and has to restart. It is then revealed to the reader, that some passersby were only trying to ask Cueball for directions to the theater, and he was just grossly overthinking it. (A recurring theme in xkcd. See: 222: Small Talk, 439: Thinking Ahead, 1643: Degrees). One can imagine Cueball having his mind in astrophysics so much that he needs to calculate the angle of the road relative to the plane of the galaxy to determine which way a destination is in conversational terms.
In the title text, Cueball mentions he has a pocket Stonehenge. During the equinoxes the sun lines up with the actual Stonehenge's pillars. Assuming you were at the actual monument, armed with the date you could calculate the cardinal directions based on the Sun's location relative to the pillars.
Transcript[edit]
- [Cueball appears to be tilted on a descending slope, with his arms held out. There is a thought bubble above his head, with the top, left and right of the bubble cut off due to its size. His thoughts are arranged into four paragraphs in the bubble.]
- Cueball (thinking): I'm facing West so the Earth's spin is carrying me backward. But our orbit is carrying me forward around the Sun.
- The Sun is passing over my left shoulder. I'm at 39°N, so I'm tilted. But wait, Earth's axis is tilted by 23°. Do I add or subtract that to get the tilt of the Solar System?
- Ok, I see the Moon. It follows the Sun's path, but is it moving toward it or away? I know it orbits counterclockwise from the North...
- My head hurts. Let me start over.
- [Two off-screen voices coming from the bottom right of the panel.]
- Off-screen voice #1: He's just standing there. Hey, do you know which way the theater is or not?
- Off-screen voice #2: Let's ask someone else.
- [Caption below the panel:]
- I spend way too much time trying to work out my orientation relative to other stuff in the universe.
Discussion
Dunno where to put this, but Captcha is giving a deprecation notice and asking to move to reCaptcha... Miguel Piedrafita 17:46, 7 March 2018 (UTC)
Someone better make a pocket stonehenge now. Linker (talk) 17:42, 7 March 2018 (UTC)
- Aren't all those pocket whatsits running on silicon close enough?
- Gene Wirchenko [email protected]
- http://www.stonehengewatch.com/ Wonder if Randall saw this before the comic...Linker (talk) 14:16, 8 March 2018 (UTC)
- http://www.iankitching.me.uk/humour/hippo/henge.html - the pocket Stonehenge made me think of this! If you want the audio, listen to the first track of https://www.youtube.com/watch?v=usdf8UHL0vU . 172.68.174.52 16:41, 8 March 2018 (UTC)
I would be remiss if I didn't mention that this comic was published two weeks before the vernal equinox 162.158.62.45 19:20, 7 March 2018 (UTC)
I started to nerd snipe myself as I tried to figure out that latitude/earth tilt thing. I have come to the conclusion that it depends on the time of year. He would be 39 degrees on the equinoxes, 16 degrees on the summer solstice, and 52 degrees on the winter solstice. I assume this is in relation to the solar system, but I know pretty much nothing about astrophysics, and I probably worded it all wrong in the first place.172.69.70.137 20:54, 7 March 2018 (UTC)
- I guess it mainly depends on the hour of the day: for example, at 12:00 solar time of the spring equinox day, the tilt would be 16 degrees ; but because of the Earth rotation, 12 hours later, it would be at 52 degrees (or 128 degrees)... 172.68.46.143
Is there a category for overly thinking things? If not, should we create one? Herobrine (talk) 23:21, 7 March 2018 (UTC)
- I don't think there is a category, but there is a word; "nerd-sniping" 108.162.216.208 01:12, 8 March 2018 (UTC)
- Do you think #1917 would be relevant for this? 162.158.126.76 12:03, 8 March 2018 (UTC)
- Yeah, someone (not me) should make one for it...Linker (talk) 14:13, 8 March 2018 (UTC)
- A couple of weeks ago
I was doing this to figure out my relative motion to the plane of the galactic (without the latitude with respect to the moon part, and lying in bed so I wouldn't fall over).Cutech (talk) 08:10, 11 March 2018 (UTC)
Perhaps Cueball needs to go live with the Kuuk Thaayorre people of Cape York in Northern Queensland. These folks don't use egocentric directions, but use cardinal dirctions for everything: "There's an ant on your southeast leg"... A good discussion is found at < https://www.edge.org/conversation/how-does-our-language-shape-the-way-we-think >. 172.68.2.64 12:06, 8 March 2018 (UTC)
Hey, when you outright delete someone's contribution, it would be great if you'd include an explanation of the edit to help support the ego of the person who wrote it =) 172.68.54.148 12:16, 8 March 2018 (UTC)
The description asserts that Cueball was overthinking his attempt to direct the out of frame person to the theatre, but that really depends on where the theatre is. If the theatre is not on Earth Cueball's reasoning could be considered relatively simplistic. 162.158.154.43 15:54, 8 March 2018 (UTC)
Suppose we want to know what the angle is between Cueball and the solar plane on the day of the spring equinox, at the time when it is solar noon at the point on the equator directly south of Cueball. We can call this point on the equator A and call Cueball’s position C. By definition, the plane of the Earth’s orbit around the sun (which we are considering to be the same as the plane of the solar system) passes through the center of the Earth. It also, at this time, passes through point A. Now, there must be some point B that is the point on Earth’s surface that is closest to Cueball while lying on the solar plane. This point is NOT necessarily point A, which is the point on Earth’s surface that is the closest to Cueball while lying on the equatorial plane.
The angle between Cueball and the solar plane should basically equal the number of degrees between Cueball and point B. We can get a rough approximation for this using the Pythagorean theorem. The Pythagorean theorem is NOT valid on the surface of a sphere when dealing with large distances relative to the size of the sphere. That is, just because the shortest arc along the surface of the sphere from point A to point B on the sphere forms a right angle with the path from B to C at B, does NOT mean you can square the great circle distance from A to B and add it to the square of the great circle distance from B to C to get the great circle distance from A to C. Nonetheless, we can use the Pythagorean theorem to get a very rough approximation.
The “line segment” (actually an arc) along Earth’s surface between A and B lies along the solar plane, since A and B are both on the solar plane. Since shortest distances are found using a perpendicular, the arc from B to C is perpendicular to this. So, A, B, and C form a sort of right triangle on the surface of the Earth. The angle between AB and AC is equal to the Earth’s orbital tilt of about 23 degrees. The distance AC is 39 degrees (that is, 39/360 of the Earth’s circumference). Since AC is the hypotenuse, the cosine of 23.4 degrees must equal BC over AC, so BC equals cos(23.4 degrees) times 39. This yields 35.8 degrees, an approximation for the angle between Cueball and the solar plane. 108.162.216.190 22:30, 10 March 2018 (UTC)
So, is the angle that Cueball is standing in the comic relative to the orientation of my monitor realistic for the angle that he's standing relative to Earth's orbital plane as described or not? davidgro (talk) 01:12, 27 March 2018 (UTC)
Okay, this explanation is ridiculously long, and explains like everything, how is it still incomplete? You can add the tag again if you want, but I think it's complete. Herobrine (talk) 07:02, 8 April 2018 (UTC)
Would it be worth mentioning that 39 degrees north latitude is roughly Washington, DC?
