Difference between revisions of "2908: Moon Armor Index"
(Replaced content with "pinis lol") |
B for brain (talk | contribs) m (→Explanation) |
||
| (26 intermediate revisions by 18 users not shown) | |||
| Line 1: | Line 1: | ||
| − | + | {{comic | |
| + | | number = 2908 | ||
| + | | date = March 18, 2024 | ||
| + | | title = Moon Armor Index | ||
| + | | image = moon_armor_index_2x.png | ||
| + | | imagesize = 740x390px | ||
| + | | noexpand = true | ||
| + | | titletext = Astronomers are a little unsure of the applicability of this index, but NASA's Planetary Protection Officer is all in favor. | ||
| + | }} | ||
| + | |||
| + | ==Explanation== | ||
| + | In this “What If?”-style comic, [[Randall]] hypothesizes an imaginative situation in which each planet's moon(s) become converted into protective armor (as a form of {{w|Overburden#Analogous uses|overburden}}) to coat the respective planet. For example, the {{w|Moon}} would coat {{w|Earth}} in a 43 kilometer layer if it were molded into protective armor, almost five times the height of {{w|Mount Everest}}. | ||
| + | |||
| + | This visual index illustrates that the moons of both Earth and Pluto are unusually massive in comparison to their planet. The large relative size of Earth’s moon — and its protective role in deflecting asteroids — is one reason that’s been suggested by astronomers for why intelligent life successfully evolved on Earth. | ||
| + | |||
| + | Mars's moons {{w|Phobos (moon)|Phobos}} and {{w|Deimos (moon)|Deimos}} are small compared to Mars, so they would contribute a thin 2-inch layer of 'armor' around Mars, in contrast to the 20-inch (0.5 m) diameter of a {{w|Mars rover}} wheel. Huge Jupiter would be covered with almost 3 km of "moon" matter, which indicates just how much moon mass orbits Jupiter, a situation mostly similar for Saturn, Uranus, and Neptune. | ||
| + | |||
| + | Six trans-Neptunian {{w|dwarf planet}}s and dwarf planet candidates are included, as well: Only Pluto, having a moon ({{w|Charon (moon)|Charon}}) of a comparable size to its planet, would have a layer thicker than Earth's. {{w|120347 Salacia|Salacia}}, {{w|Haumea}}, {{w|50000 Quaoar|Quaoar}}, {{w|225088 Gonggong|Gonggong}} and {{w|Eris (dwarf planet)|Eris}} are among the {{w|List of trans-Neptunian objects#List|ten largest such objects}}. (Two dwarf planets with moons — {{w|Makemake}} and {{w|90482 Orcus|Orcus}} — are not mentioned in the comic, but would be similarly depicted.) | ||
| + | |||
| + | The title text states that astronomers are "unsure" about the applicability of this index, a joking understatement that imagines this comic as being a serious contribution to astronomical academic knowledge. Astronomers might also point out additional issues: | ||
| + | * wariness of {{w|Giant-impact hypothesis|moons and planets getting too close}}. | ||
| + | * moons already serve a protective purpose by deflecting and even intercepting some incoming asteroids (with a ''slight'' chance of turning a future miss into a hit). | ||
| + | * the four gas giants — Jupiter, Saturn, Uranus, and Neptune — lack a solid surface to practically sustain a layer of armor without even ''more'' ambitious engineering than the already complicated process of somehow distributing soft-landed fragments of disassembled satellite evenly all across a planet. | ||
| + | * although the coating would provide some protection to the underlying surface on which it was placed, it would effectively become part of the planet, and raise the surface. The things we would normally care about protecting, such as any life forms that exist, would be forced to relocate to this new surface, and therefore not benefit from any protection, while suffering significant detrimental impact to habitats, etc. | ||
| + | |||
| + | The title text continues that NASA's [https://what-if.xkcd.com/117/ Planetary Protection Officer] is purportedly in favor of the idea. In reality, this officer is actually responsible for keeping other celestial bodies safe from Earth's contamination, not for shielding planets in armor. Theoretically, though, armoring other planets could indeed protect them from further Earth-sourced contamination, and armoring Earth would also theoretically protect other planets by burying the biosphere and all of Earth life not already sent into space — a potentially civilization-smothering action, though a surprisingly unapocalyptic result compared to many of Randall’s “What If?” scenarios. | ||
| + | |||
| + | {| class="wikitable" | ||
| + | |- | ||
| + | ! Planet/<br>dwarf planet !! Surface area (km²) || Moons || Total volume (km³) || Moon shield thickness | ||
| + | |- | ||
| + | | {{w|Earth}} || 5.1007×10^8 || {{w|Moon|1}} || 2.196×10^10 || 43 km (27 mi) (4.86 × height of {{w|Mount Everest}}) | ||
| + | |- | ||
| + | | {{w|Mars}} || 1.4437×10^8 || {{w|Moons of Mars|2}} || {{w|Phobos (moon)|(5695±32)}}+{{w|Deimos (moon)|(1033±19)}} || 5 cm (2 in) | ||
| + | |- | ||
| + | | {{w|Jupiter}} || 6.1469×10^10 || {{w|Moons of Jupiter|95}} || 1.7646×10^11 || 2.87 km (1.78 mi) | ||
| + | |- | ||
| + | | {{w|Saturn}} || 4.27×10^10 || {{w|Moons of Saturn|146}} || 7.651×10^10 || 1.79 km (1.11 mi) | ||
| + | |- | ||
| + | | {{w|Uranus}} || 8.1156×10^9 || {{w|Moons of Uranus|28}} || 5.61×10^9 || 0.69 km (0.43 mi) | ||
| + | |- | ||
| + | | {{w|Neptune}} || 7.6187×10^9 || {{w|Moons of Neptune|16}} || 1.04×10^10 || 1.36 km (0.84 mi) | ||
| + | |- | ||
| + | | {{w|Pluto}} || 1.7744×10^7 || {{w|Moons of Pluto|5}} || {{w|Charon (moon)|(9.322×10^8)}}+{{w|Moons of Pluto|(approx 87100+38800+900+200)}} || 52.5 km (32.6 mi) (by this comic's approximation) | ||
| + | 50.4 km (31.3 mi) (by full calculation) | ||
| + | |- | ||
| + | | {{w|120347 Salacia|Salacia}} || 2.27×10^6 || {{w|Actaea (moon)|1}} || 1.41×10^7 || 6.21 km (3.85 mi) | ||
| + | |- | ||
| + | | {{w|Haumea}} || 8.14×10^6 || {{w|Moons of Haumea|2}} || {{w|Hiʻiaka (moon)|(17.2×10^6)}}+{{w|Namaka (moon)|(2.57×10^6)}} || 2.43 km (1.51 mi) | ||
| + | |- | ||
| + | | {{w|50000 Quaoar|Quaoar}} || 3.78×10^6 || {{w|Weywot|1}} || 4.19×10^6 || 1.11 km (0.69 mi) | ||
| + | |- | ||
| + | | {{w|225088 Gonggong|Gonggong}} || 4.75×10^6 || {{w|Xiangliu (moon)|1}} || 1.44×10^6 || 0.3 km (0.19 mi) | ||
| + | |- | ||
| + | | {{w|Eris (dwarf planet)|Eris}} || (1.70±0.02)×10^7 || {{w|Dysnomia (moon)|1}} || 1.61×10^8 || 9.47 km (5.88 mi) | ||
| + | |} | ||
| + | |||
| + | ===Implications of choosing a volume-to-area ratio=== | ||
| + | The usual means of comparing a moon to a planet might be to compare the volume of both. This comic compares moon volume (kilometers cubed) to planet surface area (kilometers squared); specifically, the index derives a ''linear'' indicator (the thickness of the new material) by dividing the ''area'' of the main body (proportional to the square of its uncounted radius) into the ''combined volume'' of all other bodies (proportioned cubes of their own radii), which gives an unusual dimensional analysis (dividing X kilometers-cubed by Y kilometers-squared gives a length, Z, in kilometers, not a simple dimensionless ratio). | ||
| + | |||
| + | This particular methodology makes the Pluto-Charon system (Charon being roughly half the diameter and one-eighth the volume of Pluto, before even adding that of the other moons) surprisingly similar to the Earth-Moon one (our sole Moon is around one-quarter Earth's diameter, and therefore less than 2% its volume; also in comparison, the Earth and Moon are respectively slightly more than 150 times and around 3 times the volume of Pluto), but leaves them ''both'' as still standing out significantly against all other planetary comparisons, even against comparably-sized 'planet's. | ||
| + | |||
| + | ===The complexities of armor thickness calculations=== | ||
| + | The comic uses the ≈ sign to show that the formula is only an approximation: it does not take account the increase in armor surface area as it gets thicker. This approximation would be perfect for a shield of thickness zero, but for the thickest shield (Pluto) around a small celestial body the error is around 4% (52.5 km by this approximation, but 50.4 km by more thorough calculation). To find the correct value, we can use the formula for the volume of a sphere, V = 4/3 * pi * r³ (where V is the volume and r is the radius). Using this formula, we can find and add together the volumes of each moon, as well as the volume of the planet, to get a total volume of the new shielded planet. Then we can find its radius using the formula r = (V / (4/3 * pi))<sup>⅓</sup>, derived from the previous formula. Subtracting the radius of the previous planet from the radius of the new planet gives us the thickness of the armor. | ||
| + | |||
| + | This process described above assumes that all objects involved are completely spherical, which may not be the case. The act of tearing apart a solid moon, perhaps into rough gravel, might add microvoids to the new layering that bulk up the volume slightly. But neither are gravitational compression effects taken into account on an originally loose material; the planet's gravitational pull could settle some of the moon material into a slightly smaller volume than the one it occupied as lower-gravity moon. | ||
| + | |||
| + | The planet below could also be marginally affected by the change in its total planet-and-armor mass, for rocky planets mostly within any {{w|pedosphere}} or previously exposed outer {{w|lithosphere}}. The interaction with {{w|Titan (moon)#Lakes|surface liquids}} and atmospheres, especially in planets defined {{w|Gas giant|primarily by their gas layers}}, would depend much upon how impermeable and/or rigid the chosen layering method made the additional material. One could imagine a spherical shell of moon matter around Jupiter with such high structural strength as to resist crumbling into its gaseous maw. Alternatively, the moon material could be expected to sink towards the gaseous planet's center until it reaches a layer sufficiently dense and/or rigid to stop it sinking further. In this case the moon material would displace a volume of the planet's gas causing an increase in the planet's radius. | ||
| + | |||
| + | ==Transcript== | ||
| + | {{incomplete transcript|Do NOT delete this tag too soon.}} | ||
| + | :[Text above diagram:] | ||
| + | :Moon armor index: | ||
| + | :How thick the shells around various worlds would be if their moon(s) were converted into protective armor | ||
| + | :≈Total moon volume/Planet surface area | ||
| + | |||
| + | :[Above the diagram, there is a depiction of two moons orbiting a planet, an arrow pointing right, and the same planet with an additional layer around it without orbiting moons.] | ||
| + | |||
| + | :[The diagram consists of vertical bars showing "moon armor" thicknesses for the Earth, Mars, Jupiter, Saturn, Uranus, Neptune, Pluto, Salacia, Haumea, Quaoar, Gonggong and Eris. Earth's bar has a label named "43 km thick" and is compared to the height of a comparatively small Mt Everest, with randomly drawn features indicating a cross section of the additional layer's rocky material. Most of the other armor thickness bars are not very tall compared to Earth. Some bars, notably Jupiter's, are embellished with various strata-like lines that possibly correspond to different contributing moons. Most bars show some small dots and patterns. A circular viewport shows the zoomed in detail of the top of Mars's otherwise not visible bar that reveals a thin layer with the label of 2", and also the bottom of a Mars rover wheel on top of the new surface. Pluto's bar is slightly taller than Earth's and has a label "(Mostly Charon)" inside, with arrows pointing into the bar area, which looks similar to that of Earth's Moon.] | ||
| + | |||
| + | {{comic discussion}} | ||
| + | |||
| + | [[Category:Astronomy]] | ||
| + | [[Category:Bar charts]] | ||
| + | [[Category:Mars rovers]] | ||
Latest revision as of 09:30, 25 March 2024
| Moon Armor Index |
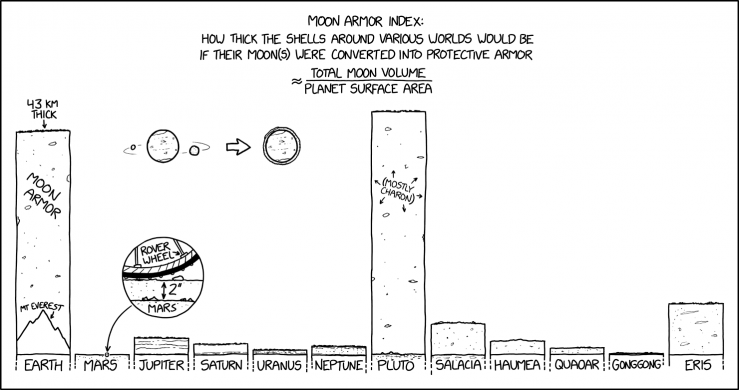 Title text: Astronomers are a little unsure of the applicability of this index, but NASA's Planetary Protection Officer is all in favor. |
Explanation[edit]
In this “What If?”-style comic, Randall hypothesizes an imaginative situation in which each planet's moon(s) become converted into protective armor (as a form of overburden) to coat the respective planet. For example, the Moon would coat Earth in a 43 kilometer layer if it were molded into protective armor, almost five times the height of Mount Everest.
This visual index illustrates that the moons of both Earth and Pluto are unusually massive in comparison to their planet. The large relative size of Earth’s moon — and its protective role in deflecting asteroids — is one reason that’s been suggested by astronomers for why intelligent life successfully evolved on Earth.
Mars's moons Phobos and Deimos are small compared to Mars, so they would contribute a thin 2-inch layer of 'armor' around Mars, in contrast to the 20-inch (0.5 m) diameter of a Mars rover wheel. Huge Jupiter would be covered with almost 3 km of "moon" matter, which indicates just how much moon mass orbits Jupiter, a situation mostly similar for Saturn, Uranus, and Neptune.
Six trans-Neptunian dwarf planets and dwarf planet candidates are included, as well: Only Pluto, having a moon (Charon) of a comparable size to its planet, would have a layer thicker than Earth's. Salacia, Haumea, Quaoar, Gonggong and Eris are among the ten largest such objects. (Two dwarf planets with moons — Makemake and Orcus — are not mentioned in the comic, but would be similarly depicted.)
The title text states that astronomers are "unsure" about the applicability of this index, a joking understatement that imagines this comic as being a serious contribution to astronomical academic knowledge. Astronomers might also point out additional issues:
- wariness of moons and planets getting too close.
- moons already serve a protective purpose by deflecting and even intercepting some incoming asteroids (with a slight chance of turning a future miss into a hit).
- the four gas giants — Jupiter, Saturn, Uranus, and Neptune — lack a solid surface to practically sustain a layer of armor without even more ambitious engineering than the already complicated process of somehow distributing soft-landed fragments of disassembled satellite evenly all across a planet.
- although the coating would provide some protection to the underlying surface on which it was placed, it would effectively become part of the planet, and raise the surface. The things we would normally care about protecting, such as any life forms that exist, would be forced to relocate to this new surface, and therefore not benefit from any protection, while suffering significant detrimental impact to habitats, etc.
The title text continues that NASA's Planetary Protection Officer is purportedly in favor of the idea. In reality, this officer is actually responsible for keeping other celestial bodies safe from Earth's contamination, not for shielding planets in armor. Theoretically, though, armoring other planets could indeed protect them from further Earth-sourced contamination, and armoring Earth would also theoretically protect other planets by burying the biosphere and all of Earth life not already sent into space — a potentially civilization-smothering action, though a surprisingly unapocalyptic result compared to many of Randall’s “What If?” scenarios.
| Planet/ dwarf planet |
Surface area (km²) | Moons | Total volume (km³) | Moon shield thickness |
|---|---|---|---|---|
| Earth | 5.1007×10^8 | 1 | 2.196×10^10 | 43 km (27 mi) (4.86 × height of Mount Everest) |
| Mars | 1.4437×10^8 | 2 | (5695±32)+(1033±19) | 5 cm (2 in) |
| Jupiter | 6.1469×10^10 | 95 | 1.7646×10^11 | 2.87 km (1.78 mi) |
| Saturn | 4.27×10^10 | 146 | 7.651×10^10 | 1.79 km (1.11 mi) |
| Uranus | 8.1156×10^9 | 28 | 5.61×10^9 | 0.69 km (0.43 mi) |
| Neptune | 7.6187×10^9 | 16 | 1.04×10^10 | 1.36 km (0.84 mi) |
| Pluto | 1.7744×10^7 | 5 | (9.322×10^8)+(approx 87100+38800+900+200) | 52.5 km (32.6 mi) (by this comic's approximation)
50.4 km (31.3 mi) (by full calculation) |
| Salacia | 2.27×10^6 | 1 | 1.41×10^7 | 6.21 km (3.85 mi) |
| Haumea | 8.14×10^6 | 2 | (17.2×10^6)+(2.57×10^6) | 2.43 km (1.51 mi) |
| Quaoar | 3.78×10^6 | 1 | 4.19×10^6 | 1.11 km (0.69 mi) |
| Gonggong | 4.75×10^6 | 1 | 1.44×10^6 | 0.3 km (0.19 mi) |
| Eris | (1.70±0.02)×10^7 | 1 | 1.61×10^8 | 9.47 km (5.88 mi) |
Implications of choosing a volume-to-area ratio[edit]
The usual means of comparing a moon to a planet might be to compare the volume of both. This comic compares moon volume (kilometers cubed) to planet surface area (kilometers squared); specifically, the index derives a linear indicator (the thickness of the new material) by dividing the area of the main body (proportional to the square of its uncounted radius) into the combined volume of all other bodies (proportioned cubes of their own radii), which gives an unusual dimensional analysis (dividing X kilometers-cubed by Y kilometers-squared gives a length, Z, in kilometers, not a simple dimensionless ratio).
This particular methodology makes the Pluto-Charon system (Charon being roughly half the diameter and one-eighth the volume of Pluto, before even adding that of the other moons) surprisingly similar to the Earth-Moon one (our sole Moon is around one-quarter Earth's diameter, and therefore less than 2% its volume; also in comparison, the Earth and Moon are respectively slightly more than 150 times and around 3 times the volume of Pluto), but leaves them both as still standing out significantly against all other planetary comparisons, even against comparably-sized 'planet's.
The complexities of armor thickness calculations[edit]
The comic uses the ≈ sign to show that the formula is only an approximation: it does not take account the increase in armor surface area as it gets thicker. This approximation would be perfect for a shield of thickness zero, but for the thickest shield (Pluto) around a small celestial body the error is around 4% (52.5 km by this approximation, but 50.4 km by more thorough calculation). To find the correct value, we can use the formula for the volume of a sphere, V = 4/3 * pi * r³ (where V is the volume and r is the radius). Using this formula, we can find and add together the volumes of each moon, as well as the volume of the planet, to get a total volume of the new shielded planet. Then we can find its radius using the formula r = (V / (4/3 * pi))⅓, derived from the previous formula. Subtracting the radius of the previous planet from the radius of the new planet gives us the thickness of the armor.
This process described above assumes that all objects involved are completely spherical, which may not be the case. The act of tearing apart a solid moon, perhaps into rough gravel, might add microvoids to the new layering that bulk up the volume slightly. But neither are gravitational compression effects taken into account on an originally loose material; the planet's gravitational pull could settle some of the moon material into a slightly smaller volume than the one it occupied as lower-gravity moon.
The planet below could also be marginally affected by the change in its total planet-and-armor mass, for rocky planets mostly within any pedosphere or previously exposed outer lithosphere. The interaction with surface liquids and atmospheres, especially in planets defined primarily by their gas layers, would depend much upon how impermeable and/or rigid the chosen layering method made the additional material. One could imagine a spherical shell of moon matter around Jupiter with such high structural strength as to resist crumbling into its gaseous maw. Alternatively, the moon material could be expected to sink towards the gaseous planet's center until it reaches a layer sufficiently dense and/or rigid to stop it sinking further. In this case the moon material would displace a volume of the planet's gas causing an increase in the planet's radius.
Transcript[edit]
| |
This transcript is incomplete. Please help editing it! Thanks. |
- [Text above diagram:]
- Moon armor index:
- How thick the shells around various worlds would be if their moon(s) were converted into protective armor
- ≈Total moon volume/Planet surface area
- [Above the diagram, there is a depiction of two moons orbiting a planet, an arrow pointing right, and the same planet with an additional layer around it without orbiting moons.]
- [The diagram consists of vertical bars showing "moon armor" thicknesses for the Earth, Mars, Jupiter, Saturn, Uranus, Neptune, Pluto, Salacia, Haumea, Quaoar, Gonggong and Eris. Earth's bar has a label named "43 km thick" and is compared to the height of a comparatively small Mt Everest, with randomly drawn features indicating a cross section of the additional layer's rocky material. Most of the other armor thickness bars are not very tall compared to Earth. Some bars, notably Jupiter's, are embellished with various strata-like lines that possibly correspond to different contributing moons. Most bars show some small dots and patterns. A circular viewport shows the zoomed in detail of the top of Mars's otherwise not visible bar that reveals a thin layer with the label of 2", and also the bottom of a Mars rover wheel on top of the new surface. Pluto's bar is slightly taller than Earth's and has a label "(Mostly Charon)" inside, with arrows pointing into the bar area, which looks similar to that of Earth's Moon.]
Discussion
Can someone hurry up/w the explanation?162.158.159.162 22:43, 18 March 2024 (UTC)
- Did it :) --1234231587678 (talk) 00:16, 19 March 2024 (UTC)
According to https://sl.bing.net/kR6wrqrekg0 it would be 43.1 meters. 172.70.174.117 23:17, 18 March 2024 (UTC)
Bing was wrong, it screwed up the units 172.70.38.181 23:39, 18 March 2024 (UTC)!
- Ew, you use Bing? Psychoticpotato (talk) 21:37, 6 May 2024 (UTC)
Anyone figure out if this takes the recently-discovered moons into account? I'd expect as much but it would make a good addition to the explanation. 172.70.131.155 01:39, 19 March 2024 (UTC)
- The new moon around Uranus is 8 km in diameter, and the moons around Neptune are 23 km and 14 km in diameter. The inventory of outer moons is believed to be complete down to 2 km for Jupiter, 3 km for Saturn, 8 km for Uranus, and 14 km for Neptune. And the total combined mass of smaller moons (e.g. in Saturn's rings) is also constrained.
- All these moons are round, and thus approximately ball-shaped. The volume of a 3-ball with radius r₀ is 4⁄3 πr₀³. Uranus and Neptune are also approximately ball-shaped with radii of 25,559 km and 15,299 km, respectively. (I don't know exactly how these radii are defined, but I assume optically. Uranus and Neptune don't have solid surfaces.) The volume of a spherical shell is just the difference of the outer and inner spheres, so 4⁄3 π(R³−r³) if the outer radius is R and the inner radius is r. These volumes are equal if the whole moon is converted into a spherical shell. So for Uranus, we have 4⁄3 πr₀³ = 4⁄3 π(R³−r³), where r₀ is the radius of the moon, r is the radius of Uranus, and R−r is the thickness of the shell. Solving gives R−r = ³√(r₀³+r³)−r. Plugging in r₀ = 8 km and r = 25,559 km gives R−r = 0.26 mm. If we laid it on top of the other moons instead of the "surface" of Uranus itself, it would make practically no difference. Doing the same calculation for each newly-discovered moon of Neptune gives thicknesses of 17 mm and 3.9 mm (for a total of 21 mm).
- In other words, they are tiny rounding errors. EebstertheGreat (talk) 03:17, 19 March 2024 (UTC)
- Not for Pluto, it seems... small planet, huge moon. Transgalactic (talk) 21:30, 19 March 2024 (UTC)
I like that turning the Moon into a spherical shell coating the Earth is not definitely stated to be impossible with current technology. There's so much hedging going on I feel like I'm trapped in a maze in The Shining. EebstertheGreat (talk) 03:17, 19 March 2024 (UTC)
The formula used seems to give the instantaneous technical distance, but in reality, there would be a rate of change of the surface area of the planet as each layer of thickness x was added. Does anyone know if this is significant with the distances we are talking, or does it just turn out to be a rounding error? 172.68.0.254 03:34, 19 March 2024 (UTC)
- For most, I suspect it is indeed the roundingest of rounding errors. Obviously, Earth+Moon and Pluto+(Charon+the others) would be the most out, but subtending difference of area at (say) sea-level radius and sea-level plus 43km doesn't sound like much to account for.
- A=4πr², so Adif of A2-A1 would be (4πr2²)-(4πr1²) or 4π(r2²-r1²) ((which looks like you could work it out as a pythogorean calculation, i.e. model a new line-length that would go at a tangent out from r1 until it hits the endpoint of the r2 radius elsewhere ... but that's probably not useful!)).
- Given Earth at a normal 6371km (between equatorial and polar radii, to simplify as a true sphere), Earth+Moon therefore 6371+43 (using figure stated by comic), that gives ...if I've done it right... now an extra 7 million km² on top of the roughly 510 million that it normally has. An increment of 5%, by the time you start spreading your arbitrarily thin final layer (so approximate back to being 2.5% extra by volume, without actually using Eebster's alternate direct shell-volume calculation or doing an integration).
- Pluto (saying 44km of layering, as slightly more than Earth's 'pile', on its far smaller radius) isn't that much more 'off'. It would increase the surface by about 8% (so says my mental arithmatic, at least) so maybe 4% more volume than a "flat surface raised up prismatically".
- (Not quite the same as "wrap a string around a tennis ball, add an inch to its length, what is its additional radius? / wrap a string around the Earth, add an inch ..." sort of thing, due to the extra dimensionality involved, but I don't feel like doing the full algebraic differentiations necessary to establish the trend of departure.).
- It certainly initially looks like the '≈'ing of the result holds fairly well under even the two most extreme examples (cases of particularly large moons-by-volume). And, at a certain point, a planet's (single largest) moon cannot be made bigger without drifting into double-planet territory (indeed, Pluto/Charon may be considered double-dwarfs!), and then, soon after, you're switching their roles around and dismantling the 'planet' (really a moon) to armour the 'moon' (now the planet). So that probably suggests we're at our limit, with twin-binary capping our one-satellite scenarios, until you get into 'busy' N-ary systems with many not-insignificant moons but somehow an identifiable 'main body' planet in the midst of them.
- I don't think "armour the Sun with all the planets (and their moons), dwarf-planets, minor-planets, random detritus, etc" will strain that relationship. Top of my head estimate is that it'd be nowhere near as high as Earth/Pluto examples, if the Oort cloud isn't oddly massive in total. But someone can correct me if I've goofed or overly hand-waved something. 172.69.195.118 06:35, 19 March 2024 (UTC)
- If you start with a ball of radius r₀, then its volume is V = 4/3πr₀³, its surface area is 4πr₀², and the derivative of its radius with respect to its volume (and thus its mass, to within a constant, roughly), is dr/dV evaluated at r₀, or 1/(4πr₀²). So a linear approximation is r = r₀ + v/(4πr₀²), where v is the added volume. On the other hand, the exact calculation is v = 4/3π(r³–r₀³), giving r = ³√(r₀³+3v/(4π)). This has the following MacLaurin series:
- r = r₀ + v/(4πr₀²) + v²/(16π²r₀⁵) + O(v³)
- The r₀⁵ in the denominator is not as high order as the v² in the numerator, so if the cube root of v is similar in size to r₀, then this is not a good approximation. But as long as the moons are collectively much less massive than the planet, then it shouldn't matter. EebstertheGreat (talk) 05:45, 23 April 2024 (UTC)
I'm glad there are at least links to them, but shouldn’t there be at least ONE sentence HERE on explainxkcd saying what the heck the last five ‘worlds’ are? I’d bet that’s what most people needing an explanation come here to find out! and all there are are links. 162.158.186.98 09:59, 19 March 2024 (UTC)
- I added a sentence about the trans-Neptunian dwarf planets. But I don't know why Randall left out Makemake, Orcus and Sedna... any hypotheses? Transgalactic (talk) 12:20, 19 March 2024 (UTC)
- I don't know this for a fact, but is it possible that those objects have no known moons to contribute any armor thickness? Ianrbibtitlht (talk) 13:06, 19 March 2024 (UTC)
- Makemake has a small moon. Orcus has a fairly large moon relative to is size, similar to Pluto. I'm slightly bitter that Salacia is here guven that astronomers don't even consider it a dwarf planet. Orcus is also much more interesting. 172.68.64.133 08:07, 20 March 2024 (UTC)
- I don't know this for a fact, but is it possible that those objects have no known moons to contribute any armor thickness? Ianrbibtitlht (talk) 13:06, 19 March 2024 (UTC)
Imagining (especially) the gas planet examples, and some sort of mechanical means (partly overlapping plates of 'moon armour', that can slide over each other, remaining gas-tight?) allowing free vertical moment, I'm wondering how much the shell could contain and actually compress the predominantly atmospheric mass below it. Not being in orbit (perhaps give it the nominal gas-cloud spin), having chosen the amount of atmosphere it sits upon it'll not really be held up by the previously uncapped atmosphere, but as it falls inwards it must eventually pressurise the volume within until it equalises against the hermetic (and magically balanced, to not crumple and fold inwards irregularly) shielding material... 172.69.194.21 16:14, 19 March 2024 (UTC)
Now, the real challenge is doing it quickly - that is, on noticing danger, armor the planet, then dearmor and rebuild the moon when danger passes. -- Hkmaly (talk) 20:00, 19 March 2024 (UTC)
I thought I was being very clever when I added the gravitational compression effects, because some tiny moons have a low density, and some of them aren't remotely as solid as the Earth's Moon because they only formed from separate rocks quite recently. But then someone applied this thought to the planet itself, where I feel (without any motivation to do the math) that such effects should be utterly negligible 5 billion years after the solar system's formative period... (though, who knows what else Pluto/Charon hold in store??) So: I'm not sure if the bit in brackets about the minuscule gravitational compression effect on the host planet should stay in the explanation. Transgalactic (talk) 21:30, 19 March 2024 (UTC)
Since as far as we currently know, there is no life on the other planets, isn't rather biocentric to suggests that the preservation of life is relevant to protecting the planet earth? (Intended as humor, if you didn't get it.) Inquirer (talk) 00:22, 20 March 2024 (UTC)
I wasn't aware that Phobos and Deimos are so tiny. Neat! 172.70.111.45 13:58, 20 March 2024 (UTC)
- Of those we know, Phobos is listed at somewhere around 80th-or-more by size (and Deimos 90th-or-more), depending upon what you count as a moon (and any more discoveries we may be making). Both smaller than Pluto's largest two-or-three satellites (Charon, if you count it as such, plus Hydra and Nix), and and a significant number of major asteroids. At some point, we're going to be more certain whether they were actually originally Mars-crossing asteroids/similar that ended up captured, or a different origin. All indeed interesting, if it piques your interest. 172.69.194.61 15:26, 20 March 2024 (UTC)
How thick would the armor be around the Sun, if the rest of the Solar System's mass, including the Oort Cloud, were used? Before it turns to plasma, that is. 172.70.39.42 18:45, 21 March 2024 (UTC)
- Let's try and use the Munrovian approximation:
- Solar surface: 6.09×1012 or 6.078×1012 km² (I get at least two different figures, depending on where on wikipedia I look)
- System Volume:
- Sun is 1.412×1018 or 1.409×1018 km³, for reference, but we don't actually count that. It contains 99.86% of the mass, but complex density pigeonholing makes that not any easy fact to derive from.
- I can add up to 2.387×1015 volume from the largest objects (down to 400km in radius), after that is extreme guesswork, even of what objects we might not know of.
- Which means that 0.17% of the system's volume (so far counted) is not in the Sun, in case you're interested.
- What we don't know enough about, I'm not sure we can easily estimate...
- Volume/Surface=393ish km
- The first 235km is Jupiter (assuming we can do this with all that gas)
- Add the other three gas giants, we get to 392km
- The next 30 bodies contribute a little over 419m, of which Earth is 178m, Venus the next 153m, Mars 27m, from then on it very quickly becomes pocket-change (4.4cm, the last on my list)
- I doubt we can do that much more with the cumulative <400km objects, and Kuiper and Oort objects (so far uncounted) might not help significantly.
- The "new Planet 9" (post-Lovell 'Planet X') might do a little bit more, if it exists. It's supposed to be Super-Earth size, by those who think it's there to be found (and, if it isn't perhaps the same missing mass (and volume) is there in a lot of snaller trans-Neptunian objects, so still worth quoting). That's perhaps 8-64 times Earth's volume, adding 1.5-11km to this particular estimate of Sun-armour.
- I'm a little surprised it was that much, actually, I expected it to be thinner just from thinking about how the Sun had so much surface to spread the planets over. But it looks like I underestimated the gas-giant contribution, until I got my hands on hard numbers. 172.71.242.175 21:25, 21 March 2024 (UTC)
