We still have 16 explanations we need to complete. All incomplete explanations are here.
Help us finish the index of What if? articles! We need to clean up & add the new YouTube videos!
2379: Probability Comparisons
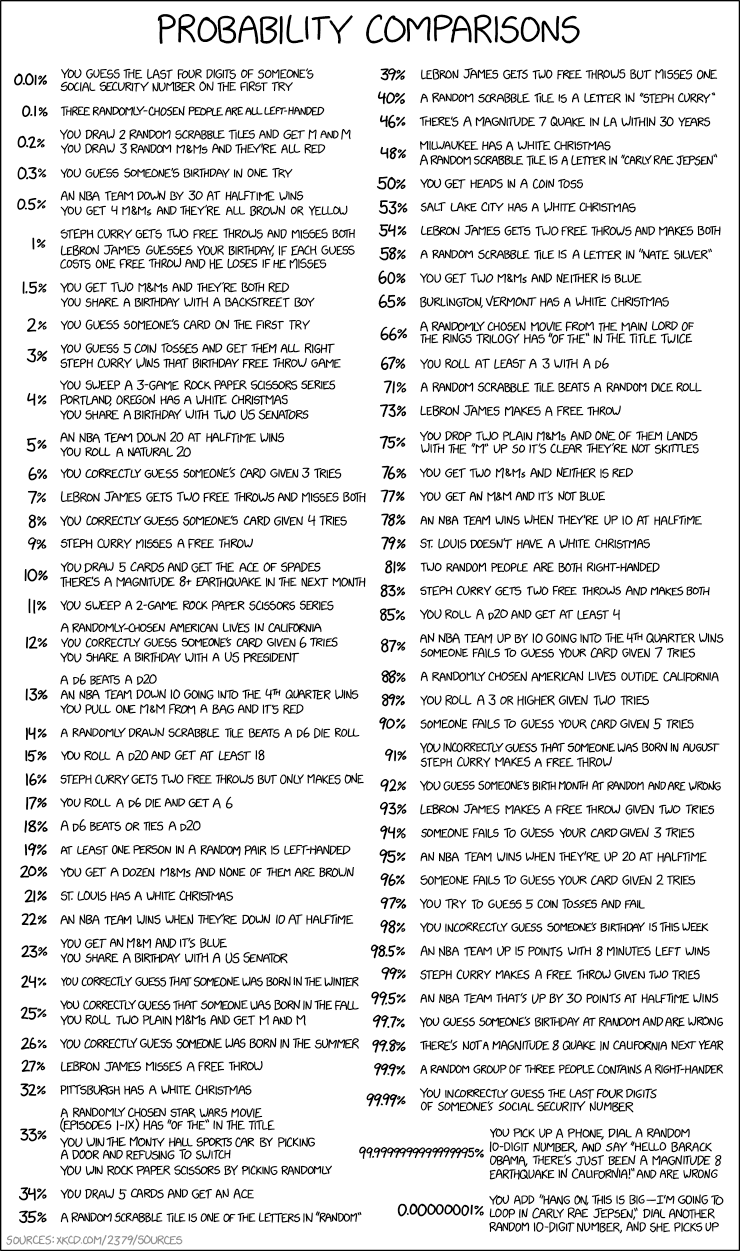
| Probability Comparisons |
 Title text: Call me, MAYBE. |
Explanation
| |
This explanation may be incomplete or incorrect: Created by LEBRON JAMES THROWING M&Ms AT A KEYBOARD. The table for the explanations of the chances isn't complete, nor is the transcript. Do NOT delete this tag too soon. If you can address this issue, please edit the page! Thanks. |
This is a list of probabilities for different events. There are numerous recurring themes, of which the most common are free throws (13 entries), birthdays (12), dice (12, split about evenly between d6 and d20 types), M&M candies (11), playing cards (9), NBA basketball mid-game victory predictions (9), Scrabble tiles (7), coins (7), white Christmases (7), and the NBA players Stephen Curry and LeBron James (7 each).
Themes are variously repeated and combined, for humorous effect. For instance, there are entries for both the probability that St. Louis will have a white Christmas (21%) and that it will not (79%). Also given is the 40% probability that a random Scrabble tile will contain a letter from the name "Steph Curry".
There are 80 items in the list, the last two of which devolve into absurdity - perhaps from the stress of preparing the other 78 entries.
The list may be an attempt to better understand probabilistic election forecasts for the 2020 United States presidential election which was less than a week away at the time this comic was published, and had also been aluded to in 2370: Prediction and 2371: Election Screen Time. Statistician and psephologist Nate Silver is referenced in one of the list items.
The probabilities are calculated from these sources, as mentioned in the bottom left corner.
| Odds | Text | Explanation | |
|---|---|---|---|
| 0.01% | You guess the last four digits of someone's Social Security Number on the first try | There are 10 digits in a Social Security Number. (1/10)4 = 0.0001, or 0.01% | |
| 0.1% | Three randomly chosen people are all left-handed | The chances of being left handed is about 10%, and 10%3 = 0.1%. | |
| 0.2% | You draw 2 random Scrabble tiles and get M and M | This appears to be an error. Under standard English Scrabble letter distribution there are 100 tiles of which 2 are M. This would give a probability of randomly drawing M and M as 2/100 × 1/99 ≈ 0.02%. However, other language editions of Scrabble have different letter distributions, some of which could allow this to be true. | |
| You draw 3 random M&Ms and they're all red | There are 3 red, 5 green, and 8 blue M&Ms. 3/16 × 2/15 × 1/14 ≈ 0.17% | ||
| 0.3% | You guess someone's birthday in one try. | 1/365 ≈ 0.27%. | |
| 0.5% | An NBA team down by 30 at halftime wins | ||
| You get 4 M&Ms and they're all brown or yellow | |||
| 1% | Steph Curry gets two free throws and misses both | ||
| LeBron James guesses your birthday, if each guess costs one free throw and he loses if he misses | |||
| 1.5% | You get two M&Ms and they're both red | ||
| You share a birthday with a Backstreet Boy | |||
| 2% | You guess someone's card on the first try | There are 52 cards in a normal deck of cards (excluding jokers), which is approximately 0.019 (2%). | |
| 3% | You guess 5 coin tosses and get them all right | The chance of correctly predicting a coin toss is 0.5. The chance of predicting 5 in a row is 0.5^5, or 3.125%. | |
| Steph Curry wins that birthday free throw game | |||
| 4% | You sweep a 3-game rock paper scissors series | ||
| Portland, Oregon has a white Christmas | |||
| You share a birthday with two US Senators | |||
| 5% | An NBA team down 20 at halftime wins | ||
| You roll a natural 20 | There are twenty sides to a d20 die. 1/20 = 0.05 = 5% | ||
| 6% | You correctly guess someone's card given 3 tries | Assuming you guess three different cards, 3/52 = 0.05769 ~ 6% | |
| 7% | LeBron James gets two free throws and misses both | ||
| 8% | You correctly guess someone's card given 4 tries | Assuming you guess four different cards, 4/52 = 0.0769 ~ 8% | |
| 9% | Steph Curry misses a free throw | ||
| 10% | You draw 5 cards and get the Ace of Spades | There are 52 cards in a normal deck of cards (excluding jokers), and the Ace of Spades is one of them. The chances of getting the card is 1 - 51/52 * 50/51 * 49/50 * 48/49 * 47/48 which is approximately 0.096, which rounds to the given 10%. | |
| There's a magnitude 8+ earthquake in the next month | |||
| 11% | You sweep a 2-game rock paper scissors series | You have a 1/3 chance of winning the first comparison, and a 1/3 chance of winning the second. (1/3) * (1/3) = 1/9 ~ 0.11 = 11% | |
| 12% | A randomly-chosen American lives in California | California is the most populous state in the U.S.A. Out of the approximately 328.2 million Americans (as of 2019), 39.51 million live in California. This means that a randomly chosen American has about a 39.51/328.2 ≈ 10.33% of being in California. Due to population change and rounding based on different sources, this could be pushed to 12%. | |
| You correctly guess someone's card given 6 tries | |||
| You share a birthday with a US President | |||
| 13% | A d6 beats a d20 | ||
| An NBA team down 10 going into the 4th quarter wins | |||
| You pull one M&M from a bag and it's red | |||
| 14% | A randomly drawn scrabble tile beats a D6 die roll | ||
| 15% | You roll a D20 and get at least 18 | ||
| 16% | Steph Curry gets two free throws but makes only one | ||
| 17% | You roll a D6 die and get a 6 | ||
| 18% | A D6 beats or ties a D20 | ||
| 19% | At least one person in a random pair is left-handed | The chances of being left handed is about 10%, so the probability of both people in the pair not being left-handed is 0.92=0.81, and 1-0.81=0.19. | |
| 20% | You get a dozen M&Ms and none of them are brown | ||
| 21% | St. Louis has a white christmas | ||
| 22% | |||
| 23% | |||
| 24% | |||
| 25% | |||
| 26% | |||
| 27% | |||
| 32% | |||
| 33% | |||
| 34% | |||
| 35% | |||
| 39% | |||
| 40% | |||
| 46% | |||
| 48% | |||
| 50% | |||
| 53% | |||
| 54% | |||
| 58% | |||
| 60% | |||
| 65% | |||
| 66% | A randomly chosen movie from the main Lord of the Rings trilogy has “of the” in the title twice | The titles are:
All of them have “of the” at least once, in “The Lord of the Rings”, but only the first and third have it twice, and 2/3 ≈ 66%. | |
| 67% | |||
| 71% | |||
| 73% | |||
| 75% | |||
| 90% | Someone fails to guess your card given 5 tries | Assuming they guess five different cards, there are 47 unguessed cards left. 47/52 = 0.90385 ~ 90% | |
| 91% | You incorrectly guess that someone was born in August | ||
| Steph Curry makes a free throw | |||
| 92% | You guess someone's birth month at random and are wrong | ||
| 93% | Lebron James makes a free throw given two tries | ||
| 94% | Someone fails to guess your card given 3 tries | ||
| 95% | An NBA team wins when they're up 20 at halftime | ||
| 96% | Someone fails to guess your card given 2 tries | ||
| 97% | You try to guess 5 coin tosses and fail | ||
| 98% | You incorrectly guess someone's birthday is this week | ||
| 98.5% | An NBA team up 15 points with 8 minutes left wins | ||
| 99% | Steph Curry makes a free throw given two tries | ||
| 99.5% | An NBA team that's up by 30 points at halftime wins | ||
| 99.7% | You guess someone's birthday at random and are wrong | ||
| 99.8% | There's not a magnitude 8 quake in California next year | ||
| 99.9% | A random group of three people contains a right-hander | ||
| 99.99% | You incorrectly guess the last four digits of someone's social security number | ||
| 99.9999999999999995% | You pick up a phone, dial a random 10-digit number, and say 'Hello Barack Obama, there's just been a magnitude 8 earthquake in California!" and are wrong | ||
| 0.00000001% | You add "Hang on, this is big — I'm going to loop in Carly Rae Jepsen", dial another random 10-digit number, and she picks up |
The title text refers to the song Call Me Maybe by Carly Rae Jepsen (cited twice in the list). "MAYBE" is emphasized perhaps because the probability of getting her phone number correct, as in the last item in the list, is very low. The capitalization could also be a reference to Scrabble tiles as was previously mentioned in association with Carly Rae Jepsen.
Trivia
In the original comic, "outside" in the 88% probability section is spelled incorrectly as "outide". In addition, the 39% section had "two free throw" instead of "throws".
Pace previous comment, the 67% probability of rolling at least a 3 with a D6 is correct. "At least a 3" means a 3, 4, 5, or 6.
Transcript
| |
This transcript is incomplete. Please help editing it! Thanks. |
PROBABILITY COMPARISONS
Discussion
(Sidenote: for the 88% entry in the comic, "outside" is misspelled as "outide" as of the current moment.)
What's the best way to organize the explanations for this comic, when they begin to be added? By the order they're listed in the comic? That seems inefficient, since presumably many of the entries can be answered as a group by a single explanation. If they should be grouped, how should they be grouped? --V2Blast (talk) 03:59, 31 October 2020 (UTC)
- The table I added is sortable. You could add a "type" column of some sort and users could sort by that if they want. Captain Video (talk) 04:42, 31 October 2020 (UTC)
There's a discrepancy between the version here and the current official version. Here, 0.2% has the red M&Ms thing paired with the odds of drawing a flush in poker ("you draw 5 cards and they're all the same suit"); the official version has it with "You draw 2 random Scrabble tiles and get M and M." Here, the latter piece of information is at 0.1%, and there the 0.1% item is "Three randomly chosen people are all left-handed." I'm guessing we have an old version of the page? Captain Video (talk) 06:03, 31 October 2020 (UTC)
- Updated. Natg19 (talk) 08:29, 31 October 2020 (UTC)
- Cool, thanks. Captain Video (talk) 01:22, 1 November 2020 (UTC)
Wouldn't the Lord of the rings one be, technically, 67%, since 66.6666666... rounds to 67%, not 66? Also, we should really add a better comment interface. BarnZarn (talk) 06:28, 31 October 2020 (UTC)
- The same goes for the next entry, imho, since LOTR-one is 2 out of 3 movies and the dice rolls are 4 out of 6, which comes down to the exact same percentage.
Hooray, xkcd is finally xkcd again! For the last fifty strips it’s basically been lighter SMBC. Yay Randall!
Also, if anyone wants to read something very English and very horrible, https://endicottstudio.typepad.com/poetrylist/the-white-road-by-neil-gaiman.html. Lightcaller (talk) 07:21, 31 October 2020 (UTC)
I have to think the second to last is off. First, what is meant by "just been"? Minutes, hours, days? Second, does anyone know the correct number of 10-digit phone numbers that are answered by people named "Barack Obama" (as pronounced, not spelled)? I remember that Obama had a cell, and including the phones in his office and his bedroom (separate #'s), so during his term, that's at least 3. SDSpivey (talk) 15:50, 31 October 2020 (UTC)
- first of all, this is no longer his term, so the number of phone numbers he has nowadays might be different. Also, the scenario requires him to pick up the phone, and he probably wouldn't simultaneously be available to pick up a phone in both his office and bedroom, and unless it's a cell phone, only a fraction of the time would he be there. Also, like many people, he might not answer calls from unknown numbers, or he may have a secretary or someone screening his calls. Judging from the following line though, the calculations used here probably just used 1 in 10 billion for that value, leaving only the "just been an 8.0 earthquake in Calfornia" part.--108.162.216.124 09:12, 1 November 2020 (UTC)
- Isn't the second to last entry really just a sneaky way of listing the probability of a magnitude 8 earthquake having just occurred in California? The entry says nothing about Barack Obama actually answering the phone, nor even that the number dialed being Barack Obama's. If agreed, then can the explanation in the table be updated? If disagreeing, then I'd appreciate you pointing out where I'm in error.
- Could Obama's phone number be referring to when he Tweeted a phone number to text him at in late September[1]? And so the chance of it being the correct number is much higher? B. A. Beder (talk) 01:09, 2 November 2020 (UTC)
guys i have never edited the transcript section im scared. — The 𝗦𝗾𝗿𝘁-𝟭 talk stalk 16:36, 31 October 2020 (UTC)
- This comic has so many American jokes and brands I can't understand this... I found this from mathematics stack exchange and that helped me understand what this M&M stuff is... — The 𝗦𝗾𝗿𝘁-𝟭 talk stalk 16:39, 31 October 2020 (UTC)
- Alright, I if the only colours are red green and blue how can there be fucking yellow or brown godammit I give up someone else do this shit AHAHAHA — The 𝗦𝗾𝗿𝘁-𝟭 talk stalk 16:45, 31 October 2020 (UTC)
- There are currently 6 colors, blue, red, brown, yellow, green and orange. Each comes in different ratios, for some reason. If there were all the same ratio, then getting 2 that are both red would be 1/36=2.777%, so red is below average. SDSpivey (talk) 00:58, 1 November 2020 (UTC)
- The colors used to be different a number of years ago. I forget what year, but they had a contest for people to vote on a new M&M flavor. They had people vote between blue, pink, and purple. I guess blue won as both pink and purple are considered girly colors and blue is considered manly, but the presencee of two girly colors split the vote for that. At the same time they got rid of there having used to be light brown M&Ms, and for a while they had commercials with blue M&Ms singing the blues. Anyway, I also read speculation the reason some colors are more common is they put less of the ones where the dye they use is more expensive, though I'm not sure if that's accurate.--108.162.216.124 09:07, 1 November 2020 (UTC)
- There are currently 6 colors, blue, red, brown, yellow, green and orange. Each comes in different ratios, for some reason. If there were all the same ratio, then getting 2 that are both red would be 1/36=2.777%, so red is below average. SDSpivey (talk) 00:58, 1 November 2020 (UTC)
- Alright, I if the only colours are red green and blue how can there be fucking yellow or brown godammit I give up someone else do this shit AHAHAHA — The 𝗦𝗾𝗿𝘁-𝟭 talk stalk 16:45, 31 October 2020 (UTC)
I don't understand the "You share a birthday with two US Senators" as being 4%. If there is only one pair of U.S. Senators with the same birthday, then your chance of sharing a birthday with them would be 1/365 (~0.27%). --162.158.74.143 20:25, 31 October 2020 (UTC)
- I'm not certain of the math offhand, but it is the odds of randomly sharing a birthday with 2 out of 100 Senators. Not that just a pair shares one with you. Although all this birthday talk ignores Feb 29 births. SDSpivey (talk) 00:58, 1 November 2020 (UTC)
- I just noticed the note about there being 9 days that have a pair of Senators sharing a birthday. Does the 4% take that into consideration? SDSpivey (talk) 01:08, 1 November 2020 (UTC)
- It's been updated to say that there are 15 days that have at least 2 Senators who share a birthday. That would make the probability (15/365.25), or 4.1%, so Randall is correct. (Using 365.25 to account for Feb. 29 births.) --162.158.74.55 03:57, 2 November 2020 (UTC)
- I just noticed the note about there being 9 days that have a pair of Senators sharing a birthday. Does the 4% take that into consideration? SDSpivey (talk) 01:08, 1 November 2020 (UTC)
Um... in the Trivia section, someone wrote:
"the 67% probability of rolling at least a 3 with a D6 is correct. "At least a 3" means a 3, 4, 5, or 6."
Four out of six is ~67%, right? Please don't tell me I've forgotten basic maths. I'm going to delete that section, but feel free to add it back in if I'm just being an idiot. BlackHat (talk) 22:28, 31 October 2020 (UTC)
The explanation for the Social Security Number is wrong- it should be that there are ten possible digits for each of the four digits you're trying to guess. The number of digits in a SSN doesn't matter since the comic specifies you're only guessing the last four. Duraludon (talk) 00:59, 1 November 2020 (UTC)
- In addition, there are no valid SSN's with any group as all zeros, so there are only 9999 valid numbers to guess at. Still close enough to .01% SDSpivey (talk) 13:21, 1 November 2020 (UTC)
XKCD comics are getting later and later in the (American) day. This one was posted Sunday the 1st, from the point of view of us Aussies. 162.158.119.159 01:40, 1 November 2020 (UTC)
This comic is how I found out I share a birthday with one of the Backstreet Boys (Nick Carter). Thanks, Randall. 172.70.126.69 23:53, 27 July 2021 (UTC)
2/3 = both 66% and 67%?
I get picking either 66% or 67% as a rounding for 2/3 but to have one of each?? Is there any actual reason for this?
66% A randomly chosen movie from the main Lord of the Rings trilogy has “of the” in the title twice
67% You roll at least a 3 with a d6
162.158.79.152 21:40, 31 October 2020 (UTC)
I wonder what time frame he meant for there "just" having been an earthquake in California.--108.162.216.124 09:03, 1 November 2020 (UTC)
Angus King is from Maine, that’s ME not MN. 108.162.219.200 14:43, 1 November 2020 (UTC)
Do we do calculus?
I think I've got how Randall did the birthday party/free-throw calculations, but it's kind of math-intensive. How much should I put in the explanation column? It's quite easier to explain with summations, but that requires a lot of background to someone who doesn't know calculus (i.e., probably a lot of people who read this). Should I forego the sum entirely? Should I say "the proof is by magic"? Also, at least some of this is stemming from the fact that I have no clue how one would insert a summation sigma into the editing, and I'm too afraid to try it. I'll write it with a bunch of plus signs (basically a sum, but longhand notation) until somebody decides to step in and clean it up. BlackHat (talk) 18:05, 1 November 2020 (UTC)
Let's talk M&Ms
I'm beginning to think Randall is nerd-sniping us, because none of the values for M&M colours seem to line up with his source. The easiest example to demonstrate is '77% : An M&M is not blue'. Nowhere in the article is there a value which rounds to 23% for blue M&Ms. Most of the other calculations also seem to have small-scale differences, and a few have differences so big only using the 95% confidence interval values help. Can anybody figure out his line of reasoning with this? BlackHat (talk) 19:12, 1 November 2020 (UTC)
- You have to remember that 87% of all stats are made up. SDSpivey (talk) 21:24, 1 November 2020 (UTC)
- The source in question does show about 23% for blue M&Ms. In 2008: 24%. In 2017, Cleveland plant: 20.7%, Hackettstown plant: 25% (average 22.85%, assuming both factories produce the same volume).108.162.229.54 13:55, 2 November 2020 (UTC)
Hemispheres and Seasons
Should there be a note of the fact that the summer/winter percentages are only true in the northern hemisphere? In the southern hemisphere, where summer is December-February and winter is June-August, the figures should be reversed (and at the equator, summer and winter don't really exist). 172.68.86.114 21:49, 1 November 2020 (UTC)
- I'm not entirely sure which season boundaries are being espoused. Equinox/Solstice ones (summer starts on "mid-summer's day", sic), mid-way between adjacent equinoces/solstices (mid-summer's day is exactly half way through summer), meteorlogical (groupings of three calendar months)..? I suspect the latter, to provide the off-quarter values from almost continually variable month-lengths, but the other two (in conjunction with the elliptical orbit of the Earth changing the rate each phase of oscillation made by the ecliptic) would be a far more scientific reason worthy of Randall. 162.158.155.102 02:47, 2 November 2020 (UTC)
- By my reckoning the proportions of seasons by various standards are as follows:
| Season | Meteorological | Summer starts 'mid-summer' | Summer astride 'mid-summer' | |||||
|---|---|---|---|---|---|---|---|---|
| Northern | Southern | Starts | Prop | Starts | Prop | Starts | Mid-point 'drift' | Prop |
| Winter 19/20 | Summer 19/20 | 1/Dec/2019 | 24.86% | 22/Dec/2019 04:19 | 24.36% | 7/Nov/2019 06:04 | 5h14m early | not calculated |
| Spring 20 | Autumn 20 | 1/Mar/2020 | 25.14% | 20/Mar/2020 03:50 | 25.39% | 4/Feb/2020 16:04 | 22h35m late | 24.88% |
| Summer 20 | Winter 20 | 1/Jun/2020 | 25.14% | 20/Jun/2020 21:43 | 25.64% | 5/May/2020 12:46 | 5h26m late | 25.52% |
| Autumn 20 | Spring 20 | 1/Sep/2020 | 24.86% | 22/Sep/2020 13:21 | 24.60% | 6/Aug/2020 17:32 | 22h44m early | 25.12% |
| Winter 20/21 | Summer 20/21 | 1/Dec/2020 | 24.66% | 21/Dec/2020 10:03 | 24.36% | 6/Nov/2020 11:42 | 5h17m early | 24.48% |
| Spring 21 | Autumn 21 | 1/Mar/2021 | 25.21% | 20/Mar/2021 09:37 | 25.39% | 3/Feb/2021 11:42 | 22h35m late | 24.88% |
| Summer 21 | Winter 21 | 1/Jun/2021 | 25.21% | 21/Jun/2021 03:32 | 25.64% | 5/May/2021 18:34 | 5h28m late | 25.52% |
| Autumn 21 | Spring 21 | 1/Sep/2021 | 24.93% | 22/Sep/2021 19:21 | 24.60% | 6/Aug/2021 23:26 | 22h47m early | 25.12% |
| Winter 21/22 | Summer 21/22 | 1/Dec/2021 | 24.66% | 21/Dec/2021 15:59 | not calc. | 6/Nov/2021 23:26 | 5h16m early | 24.48% |
- This covers two entire years (leap and non-leap). It assigns (northern) winter to whatever year it most lies within, for percentile purposes, as indicated by shared background. The 'astride' seasons start at the calculated mid-point between astronomical 'quarter-points', which is probably not how it's based IRL, and I give the mid-point difference from the quarter-point that should be their mid-point. Times are UTC, bare dates can be assumed midnight to midnight. Any leap-seconds I may have ignored are well below my level of precision. Also note E&OE, with plenty of possible transfer errors in plugging the raw details into the spreadsheet then re-transfering the spreadsheet into a wikitable format (across various screens/machines, because I'm an idiot). Also does not take into account actual demographic distribution across the solar year, which probably is what really is at work here. But I too thought it'd be interesting to look at it this way. Enjoy! 141.101.98.52 15:42, 2 November 2020 (UTC)
Obama earthquake probability
I'm was thinking about the second-to-last probability. This should be Pr[call Obama] * Pr[Magnitude 8 earthquake "just" occured in CA] = 5e-18.
- From the phrasing we assume 10-digit numbers are dialed randomly, giving Pr[call Obama] = 1e-10
- From the previous quake we know Pr[CA quake/year] = 2e-3
- The time period for "just occurred" is not defined.
- SDSpivey points out there is some ambiguity with the number of phones Obama has and whether to include the probability of him answering personally
If we assume Obama answers a single phone number than the time period would be 5e-18/(1e-10 * 2e-3) = 2.5e-5 years = 13 minutes.
It seems likely that a 15 min period was considered for "just occurred", which would be within rounding error of the quake probability. --Quantum7 (talk) 09:59, 2 November 2020 (UTC)
Free Throw meaning
Hi! Would it be possible to add an explanation as to what a free throw is, for the benefit of those of us who know nothing about basketball? Thanks! 162.158.158.183 13:03, 2 November 2020 (UTC) Sure: when one of a number of transgressions of the rules occurs (a "foul"), depending on about 17 other variables, the player who was fouled is allowed to stand at a special line called the "Free-throw line" and take either one, two or three shots at the basket without anyone guarding him. Free throws only count one point, as opposed to baskets made during play which are 2 points (or 3 points outside yet another circular arc some distance from the goal).
- Thanks. Would it be possible to include that in the main explanation text, or at least include a wikilink to an explanation? Not everyone who reads xkcd will know what one is. 162.158.159.46 12:36, 9 November 2020 (UTC)
Fairly certain this calculation is wrong. It assumes that births are divided evenly across the dates of the year, but some birth dates are more common than others. 162.158.134.84 20:59, 2 November 2020 (UTC)
- Are you referring to the fact that Feb 29 is far less common than other birthdays? Or the fact that December 25th is noticeably less common (with a similar albeit smaller uptick on Dec. 26) https://www.panix.com/~murphy/bday.html (a study of ~400,000 birth dates) and my own personal investigation using a dataset of a half million college applicants show that the distribution of birthdates is very close to the expected value that statistics would predict, with the glaring exception of Dec. 25 and 26. For the single-digit accuracy that Randal is using (rounding 2/3 to be 67% for example) the distribution of birthdays is close enough to flat for the computed value to be valid.162.158.79.87 05:15, 4 November 2020 (UTC)
